Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Jiang, Hongyi
Cheng, Liang
Draper, Scott
and
An, Hongwei
2017.
Three-dimensional wake transition for a circular cylinder near a moving wall.
Journal of Fluid Mechanics,
Vol. 818,
Issue. ,
p.
260.
Rao, Anirudh
Leontini, Justin S.
Thompson, Mark C.
and
Hourigan, Kerry
2017.
Three-dimensionality of elliptical cylinder wakes at low angles of incidence.
Journal of Fluid Mechanics,
Vol. 825,
Issue. ,
p.
245.
Jiang, Hongyi
and
Cheng, Liang
2017.
Strouhal–Reynolds number relationship for flow past a circular cylinder.
Journal of Fluid Mechanics,
Vol. 832,
Issue. ,
p.
170.
Ng, Zhi Y.
Vo, Tony
and
Sheard, Gregory J.
2018.
Stability of the wakes of cylinders with triangular cross-sections.
Journal of Fluid Mechanics,
Vol. 844,
Issue. ,
p.
721.
Jiang, Hongyi
Cheng, Liang
and
An, Hongwei
2018.
Three-dimensional wake transition of a square cylinder.
Journal of Fluid Mechanics,
Vol. 842,
Issue. ,
p.
102.
Hanif, Rabia
Bhatti, O.G.
Ebrahem, M.
Manzoor, S.
Ali, Muzaffar
and
Sheikh, N.A.
2018.
Transient fluid flow and heat transfer over a rotating circular cylinder near a wall subject to a single gust impulse.
International Journal of Heat and Mass Transfer,
Vol. 126,
Issue. ,
p.
1178.
Tanweer, Sartaj
Dewan, Anupam
and
Sanghi, Sanjeev
2019.
Influence of wake confinement and buoyancy on flow past a square cylinder.
Fluid Dynamics Research,
Vol. 51,
Issue. 3,
p.
035502.
Zhu, Hongjun
and
Zhou, Tongming
2019.
Flow around a circular cylinder attached with a pair of fin-shaped strips.
Ocean Engineering,
Vol. 190,
Issue. ,
p.
106484.
Zhu, Hongjun
Zhao, Honglei
and
Zhou, Tongming
2019.
Direct numerical simulation of flow over a slotted cylinder at low Reynolds number.
Applied Ocean Research,
Vol. 87,
Issue. ,
p.
9.
Ouro, Pablo
Muhawenimana, Valentine
and
Wilson, Catherine A. M. E.
2019.
Asymmetric wake of a horizontal cylinder in close proximity to a solid boundary for Reynolds numbers in the subcritical turbulence regime.
Physical Review Fluids,
Vol. 4,
Issue. 10,
Wang, Rui
Zhu, Hongbo
Bao, Yan
Zhou, Dai
Ping, Huan
Han, Zhaolong
and
Xu, Hui
2019.
Modification of three-dimensional instability in the planar shear flow around two circular cylinders in tandem.
Physics of Fluids,
Vol. 31,
Issue. 10,
Zhu, Hongjun
Zhang, Chun
and
Liu, Wenli
2019.
Wake-induced vibration of a circular cylinder at a low Reynolds number of 100.
Physics of Fluids,
Vol. 31,
Issue. 7,
Zhu, Hongjun
Tang, Tao
Zhao, Honglei
and
Gao, Yue
2019.
Control of vortex-induced vibration of a circular cylinder using a pair of air jets at low Reynolds number.
Physics of Fluids,
Vol. 31,
Issue. 4,
Zhang, P. J.
Lin, J. Z.
and
Ku, X. K.
2019.
Flow of power-law fluid past a circular cylinder in the vicinity of a moving wall.
Journal of the Brazilian Society of Mechanical Sciences and Engineering,
Vol. 41,
Issue. 1,
Zhu, Hongjun
and
Wang, Kenan
2019.
Wake adjustment and vortex-induced vibration of a circular cylinder with a C-shaped plate at a low Reynolds number of 100.
Physics of Fluids,
Vol. 31,
Issue. 10,
Ju, Xiaoying
An, Hongwei
Cheng, Liang
and
Tong, Feifei
2020.
Modes of synchronisation around a near-wall oscillating cylinder in streamwise directions.
Journal of Fluid Mechanics,
Vol. 893,
Issue. ,
Jiang, Hongyi
2020.
Separation angle for flow past a circular cylinder in the subcritical regime.
Physics of Fluids,
Vol. 32,
Issue. 1,
Zhu, Hongjun
Tang, Tao
Zhou, Tongming
Liu, Hongye
and
Zhong, Jiawen
2020.
Flow structures around trapezoidal cylinders and their hydrodynamic characteristics: Effects of the base length ratio and attack angle.
Physics of Fluids,
Vol. 32,
Issue. 10,
Tanweer, Sartaj
Dewan, Anupam
and
Sanghi, Sanjeev
2020.
Influence of three-dimensional wake transition on heat transfer from a square cylinder near a moving wall.
International Journal of Heat and Mass Transfer,
Vol. 148,
Issue. ,
p.
118986.
Jiang, Hongyi
and
Cheng, Liang
2020.
Transition to chaos in the cylinder wake through the Mode C flow.
Physics of Fluids,
Vol. 32,
Issue. 1,
 $G^{\ast }$) between 0.1 to 19.5 and Reynolds number (
$G^{\ast }$) between 0.1 to 19.5 and Reynolds number (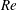 $Re$) up to 300. Variations in the flow characteristics with
$Re$) up to 300. Variations in the flow characteristics with 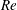 $Re$ and
$Re$ and 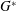 $G^{\ast }$ are studied, and their correlations with the hydrodynamic forces on the cylinder are investigated. It is also found that the monotonic increase of the critical
$G^{\ast }$ are studied, and their correlations with the hydrodynamic forces on the cylinder are investigated. It is also found that the monotonic increase of the critical 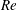 $Re$ for 2D instability (
$Re$ for 2D instability (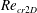 $Re_{cr2D}$) with decreasing
$Re_{cr2D}$) with decreasing 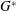 $G^{\ast }$ is influenced by variations in the mean flow rate around the cylinder, the confinement of the near-wake flow by the plane wall and the characteristics of the shear layer formed above the moving wall directly below the cylinder. The first factor destabilizes the wake flow at a moderate
$G^{\ast }$ is influenced by variations in the mean flow rate around the cylinder, the confinement of the near-wake flow by the plane wall and the characteristics of the shear layer formed above the moving wall directly below the cylinder. The first factor destabilizes the wake flow at a moderate 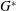 $G^{\ast }$ while the latter two factors stabilize the wake flow with decreasing
$G^{\ast }$ while the latter two factors stabilize the wake flow with decreasing 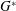 $G^{\ast }$. In terms of 3D instability, the flow transition sequence of ‘2D steady
$G^{\ast }$. In terms of 3D instability, the flow transition sequence of ‘2D steady  $\rightarrow$ 3D steady
$\rightarrow$ 3D steady  $\rightarrow$ 3D unsteady’ for small gap ratios is analysed at
$\rightarrow$ 3D unsteady’ for small gap ratios is analysed at 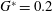 $G^{\ast }=0.2$. It is found that the 3D steady and 3D unsteady flows are triggered by Mode C instability due to wall proximity. However, the Mode C structure is not sustained indefinitely, since interference with the shear layer leads to other 3D steady and unsteady flow structures.
$G^{\ast }=0.2$. It is found that the 3D steady and 3D unsteady flows are triggered by Mode C instability due to wall proximity. However, the Mode C structure is not sustained indefinitely, since interference with the shear layer leads to other 3D steady and unsteady flow structures.