Article contents
Turbulence modulation in buoyancy-driven bubbly flows
Published online by Cambridge University Press: 03 December 2021
Abstract
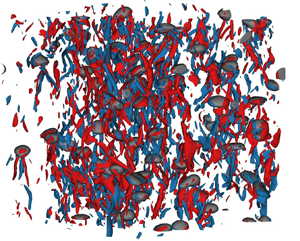
We present a direct numerical simulation (DNS) study of buoyancy-driven bubbly flows in the presence of large-scale driving that generates turbulence. On increasing the turbulence intensity: (a) the bubble trajectories become more curved and (b) the average rise velocity of the bubbles decreases. We find that the energy spectrum of the flow shows a pseudo-turbulence scaling for length scales smaller than the bubble diameter and a Kolmogorov scaling for scales larger than the bubble diameter. We conduct a scale-by-scale energy budget analysis to understand the scaling behaviour observed in the spectrum. Although our bubbles are weakly buoyant, the statistical properties of our DNS are consistent with the experiments that investigate turbulence modulation by air bubbles in water.
Information
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2021. Published by Cambridge University Press
References
REFERENCES
- 9
- Cited by


