Article contents
Turbulence at the Lee bound: maximally non-normal vortex filaments and the decay of a local dissipation rate
Published online by Cambridge University Press: 24 October 2019
Abstract
This paper uses a tight mathematical bound on the degree of the non-normality of the turbulent velocity gradient tensor to classify flow behaviour within vortical regions (where the eigenvalues of the tensor contain a conjugate pair). Structures attaining this bound are preferentially generated where enstrophy exceeds total strain and there is a positive balance between strain production and enstrophy production. Lagrangian analysis of homogeneous, isotropic turbulence shows that attainment of this bound is associated with relatively short durations and an upper limit to the spatial extent of the flow structures that is similar to the Taylor scale. An analysis of the dynamically relevant terms using a recently developed formulation (Keylock, J. Fluid Mech., vol. 848, 2018, pp. 876–904), highlights the controls on this dynamics. In particular, in high enstrophy regions it is shown that the bound is attained when normal strain decreases rather than when non-normality increases. The near absence of normal total strain results in a source of intermittency in the dynamics of dissipation that is hidden in standard analyses. It is shown that of the two terms that contribute to the non-normal production dynamics, it is the one that is typically smallest in magnitude that is of greatest importance within these 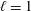 $\ell =1$ filaments. The typical distance between filament centroids is just less than a Taylor scale, implying a connection to the manner in which flow topology at the Taylor scale explains dissipation at smaller scales.
$\ell =1$ filaments. The typical distance between filament centroids is just less than a Taylor scale, implying a connection to the manner in which flow topology at the Taylor scale explains dissipation at smaller scales.
Information
- Type
- JFM Papers
- Information
- Copyright
- © 2019 Cambridge University Press
References
- 6
- Cited by


