Article contents
Superhydrophobic surface immobilisation by insoluble surfactant
Published online by Cambridge University Press: 28 September 2022
Abstract
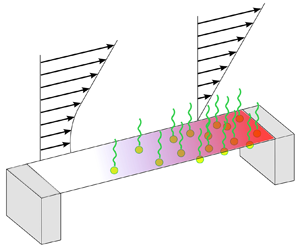
The effects of insoluble surfactants, satisfying a Langmuir equation of state, on transverse Stokes flow over a superhydrophobic surface of unidirectional grooves with flat menisci are examined. The phenomenon of surface immobilisation, whereby surfactants cause part or all of the Cassie-state menisci to become effectively no-slip zones thereby degrading the slip properties of the surface, is of primary interest. The study employs a combined analytical and numerical approach allowing an exploration of surfactant effects over the full range of surface Péclet numbers, Marangoni numbers and surfactant loads. For small surface Péclet and Marangoni numbers, perturbation theory is used to gain basic insights into the physical mechanisms at work. That analysis also provides checks on a robust numerical scheme, built around a complex variable formulation of the problem, used to compute solutions across a wide range of non-dimensional parameter values. Two distinct mechanisms are found to be responsible for surface immobilisation. In the first, most commonly seen at higher surface Péclet numbers, a stagnant cap forms because swept surfactant immobilises a section of the meniscus before any portion of the meniscus reaches maximum packing. Such a cap exists even for a linear equation of state and grows in length with increasing Marangoni number. A second immobilisation mechanism is associated with the nonlinear equation of state: an immobilised region forms because the surfactant concentration reaches its maximum value near the downstream edge of the meniscus. Immobilisation of the latter form can occur at much lower surface Péclet numbers, even if the Marangoni number is small, as long as there is sufficient surfactant in the system. The study enhances understanding of how insoluble surfactants can degrade slip over superhydrophobic surfaces.
Information
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2022. Published by Cambridge University Press
References
REFERENCES
- 10
- Cited by


