Article contents
Spin-down in rotating Hagen–Poiseuille flow: a simple criterion to detect the onset of absolute instabilities
Published online by Cambridge University Press: 16 March 2016
Abstract
We conduct experiments in a circular pipe with rotating Hagen–Poiseuille flow (RHPF) to which we apply spin-down or impulsive spin-down to rest, in order to analyse the threshold between convective and absolute instabilities through flow visualisations in the inlet region of the pipe. For a constant value of the Reynolds number,  $Re$ , the finite-amplitude wave packets generated by the arbitrary perturbation that results by reducing the swirl parameter, propagate upstream or downstream depending on the initial value of the swirl parameter,
$Re$ , the finite-amplitude wave packets generated by the arbitrary perturbation that results by reducing the swirl parameter, propagate upstream or downstream depending on the initial value of the swirl parameter,  $L_{0}$ . In fact, the main characteristic of the flow is that the velocity front of these wave packets changes from negative to positive when absolutely unstable modes are present in the initial state. The experimental results show that spin-down becomes a precise, reliable procedure to detect the onset of absolute instabilities. In addition, we give evidence of a gradual transition for Reynolds numbers ranging from 300 to 500 where a mode shift from
$L_{0}$ . In fact, the main characteristic of the flow is that the velocity front of these wave packets changes from negative to positive when absolutely unstable modes are present in the initial state. The experimental results show that spin-down becomes a precise, reliable procedure to detect the onset of absolute instabilities. In addition, we give evidence of a gradual transition for Reynolds numbers ranging from 300 to 500 where a mode shift from 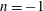 $n=-1$ to
$n=-1$ to 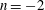 $n=-2$ appears in the absolutely unstable region.
$n=-2$ appears in the absolutely unstable region.
JFM classification
Information
- Type
- Papers
- Information
- Copyright
- © 2016 Cambridge University Press
References
Miranda-Barea et al. supplementary movie
Spin-down in rotating Hagen-Poiseuille flow is applied to detect by means of direct observation the onset of absolute instabilities. In this video, and for a constant Reynolds number of 250, we reduce suddenly the angular velocity to 50 %. The flow is convectively and absolutely unstable in the first and second sequences, respectively, thus producing different flow patterns in the temporal evolution of the perturbation-produced wave packet fronts in the pipe inlet region.
- 5
- Cited by


