Article contents
Spin-down in a rapidly rotating cylinder container with mixed rigid and stress-free boundary conditions
Published online by Cambridge University Press: 30 March 2017
Abstract
A comprehensive study of the classical linear spin-down of a constant-density viscous fluid (kinematic viscosity  $\unicode[STIX]{x1D708}$ ) rotating rapidly (angular velocity
$\unicode[STIX]{x1D708}$ ) rotating rapidly (angular velocity 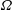 $\unicode[STIX]{x1D6FA}$ ) inside an axisymmetric cylindrical container (radius
$\unicode[STIX]{x1D6FA}$ ) inside an axisymmetric cylindrical container (radius 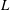 $L$ , height
$L$ , height 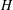 $H$ ) with rigid boundaries, which follows the instantaneous small change in the boundary angular velocity at small Ekman number
$H$ ) with rigid boundaries, which follows the instantaneous small change in the boundary angular velocity at small Ekman number  $E=\unicode[STIX]{x1D708}/H^{2}\unicode[STIX]{x1D6FA}\ll 1$ , was provided by Greenspan & Howard (J. Fluid Mech., vol. 17, 1963, pp. 385–404). For that problem
$E=\unicode[STIX]{x1D708}/H^{2}\unicode[STIX]{x1D6FA}\ll 1$ , was provided by Greenspan & Howard (J. Fluid Mech., vol. 17, 1963, pp. 385–404). For that problem  $E^{1/2}$ Ekman layers form quickly, triggering inertial waves together with the dominant spin-down of the quasi-geostrophic (QG) interior flow on the
$E^{1/2}$ Ekman layers form quickly, triggering inertial waves together with the dominant spin-down of the quasi-geostrophic (QG) interior flow on the  $O(E^{-1/2}\unicode[STIX]{x1D6FA}^{-1})$ time scale. On the longer lateral viscous diffusion time scale
$O(E^{-1/2}\unicode[STIX]{x1D6FA}^{-1})$ time scale. On the longer lateral viscous diffusion time scale 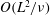 $O(L^{2}/\unicode[STIX]{x1D708})$ , the QG flow responds to the
$O(L^{2}/\unicode[STIX]{x1D708})$ , the QG flow responds to the 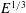 $E^{1/3}$ sidewall shear layers. In our variant, the sidewall and top boundaries are stress-free, a set-up motivated by the study of isolated atmospheric structures such as tropical cyclones or tornadoes. Relative to the unbounded plane layer case, spin-down is reduced (enhanced) by the presence of a slippery (rigid) sidewall. This is evidenced by the QG angular velocity,
$E^{1/3}$ sidewall shear layers. In our variant, the sidewall and top boundaries are stress-free, a set-up motivated by the study of isolated atmospheric structures such as tropical cyclones or tornadoes. Relative to the unbounded plane layer case, spin-down is reduced (enhanced) by the presence of a slippery (rigid) sidewall. This is evidenced by the QG angular velocity,  $\unicode[STIX]{x1D714}^{\star }$ , evolution on the
$\unicode[STIX]{x1D714}^{\star }$ , evolution on the 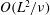 $O(L^{2}/\unicode[STIX]{x1D708})$ time scale: spatially,
$O(L^{2}/\unicode[STIX]{x1D708})$ time scale: spatially, 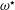 $\unicode[STIX]{x1D714}^{\star }$ increases (decreases) outwards from the axis for a slippery (rigid) sidewall; temporally, the long-time
$\unicode[STIX]{x1D714}^{\star }$ increases (decreases) outwards from the axis for a slippery (rigid) sidewall; temporally, the long-time 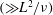 $(\gg L^{2}/\unicode[STIX]{x1D708})$ behaviour is dominated by an eigensolution with a decay rate slightly slower (faster) than that for an unbounded layer. In our slippery sidewall case, the
$(\gg L^{2}/\unicode[STIX]{x1D708})$ behaviour is dominated by an eigensolution with a decay rate slightly slower (faster) than that for an unbounded layer. In our slippery sidewall case, the 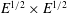 $E^{1/2}\times E^{1/2}$ corner region that forms at the sidewall intersection with the rigid base is responsible for a
$E^{1/2}\times E^{1/2}$ corner region that forms at the sidewall intersection with the rigid base is responsible for a 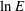 $\ln E$ singularity within the
$\ln E$ singularity within the 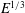 $E^{1/3}$ layer, causing our asymptotics to apply only at values of
$E^{1/3}$ layer, causing our asymptotics to apply only at values of 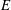 $E$ far smaller than can be reached by our direct numerical simulation (DNS) of the linear equations governing the entire spin-down process. Instead, we solve the
$E$ far smaller than can be reached by our direct numerical simulation (DNS) of the linear equations governing the entire spin-down process. Instead, we solve the 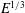 $E^{1/3}$ boundary layer equations for given
$E^{1/3}$ boundary layer equations for given 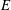 $E$ numerically. Our hybrid asymptotic–numerical approach yields results in excellent agreement with our DNS.
$E$ numerically. Our hybrid asymptotic–numerical approach yields results in excellent agreement with our DNS.
Information
- Type
- Papers
- Information
- Copyright
- © 2017 Cambridge University Press
References
- 3
- Cited by


