Published online by Cambridge University Press: 27 August 2020
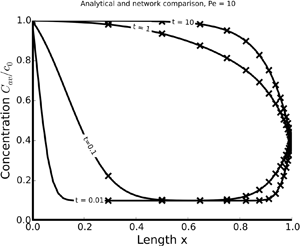
Advective–diffusive transport of passive scalars in confined environments (e.g. vessels and channels) within a network is of fundamental importance in a plethora of biological and geophysical phenomena. We conduct a leading-order analysis, consistent with the theory of hydrodynamic dispersion, which averages out the radial variability within a vessel. One-dimensional solutions for individual vessels (edges of a network), obtained for arbitrary (undefined) transient Dirichlet boundary conditions, serve as a building block for a network model. A network transport solution is developed by iteratively linking single-vessel solutions to each other in a bifurcating fractal tree model, i.e. at nodes of the network. We find transport behaviour to be strongly affected by the network geometry and daughter vessels to significantly impact the rate of transport in the upstream parent vessels.