Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Yang, Xiaobin
Liu, Cheng
Wan, Decheng
and
Hu, Changhong
2021.
Numerical study of the shock wave and pressure induced by single bubble collapse near planar solid wall.
Physics of Fluids,
Vol. 33,
Issue. 7,
Dockar, Duncan
Gibelli, Livio
and
Borg, Matthew K.
2021.
Shock-induced collapse of surface nanobubbles.
Soft Matter,
Vol. 17,
Issue. 28,
p.
6884.
Ma, Wentao
Zhao, Xuning
Gilbert, Christine
and
Wang, Kevin
2022.
Computational analysis of bubble–structure interactions in near-field underwater explosion.
International Journal of Solids and Structures,
Vol. 242,
Issue. ,
p.
111527.
Seyedmirzaei Sarraf, Seyedali
Rokhsar Talabazar, Farzad
Namli, Ilayda
Maleki, Mohammadamin
Sheibani Aghdam, Araz
Gharib, Ghazaleh
Grishenkov, Dmitry
Ghorbani, Morteza
and
Koşar, Ali
2022.
Fundamentals, biomedical applications and future potential of micro-scale cavitation-a review.
Lab on a Chip,
Vol. 22,
Issue. 12,
p.
2237.
Cao, Shunxiang
and
Zhengyu Huang, Daniel
2022.
Bayesian calibration for large‐scale fluid structure interaction problems under embedded/immersed boundary framework.
International Journal for Numerical Methods in Engineering,
Vol. 123,
Issue. 8,
p.
1791.
Nguyen, Van-Tu
Phan, Thanh-Hoang
and
Park, Warn-Gyu
2023.
Modeling of shock wave produced by collapse of cavitation bubble using a fully conservative multiphase model.
Physics of Fluids,
Vol. 35,
Issue. 11,
Xiang, Gaoming
Li, Daiwei
Chen, Junqin
Mishra, Arpit
Sankin, Georgy
Zhao, Xuning
Tang, Yuqi
Wang, Kevin
Yao, Junjie
and
Zhong, Pei
2023.
Dissimilar cavitation dynamics and damage patterns produced by parallel fiber alignment to the stone surface in holmium:yttrium aluminum garnet laser lithotripsy.
Physics of Fluids,
Vol. 35,
Issue. 3,
Аганин, А. А.
Хисматуллина, Н. А.
and
Нигматулин, Р. И.
2023.
Импульсное воздействие на коллапс кавитационного пузырька.
Теплофизика высоких температур,
Vol. 61,
Issue. 3,
p.
419.
Aganin, A. A.
Khismatullina, N. A.
and
Nigmatulin, R. I.
2023.
Pulse Impact on Cavitation Bubble Collapse.
High Temperature,
Vol. 61,
Issue. 3,
p.
385.
Zhao, Xuning
Ma, Wentao
and
Wang, Kevin
2023.
Simulating laser-fluid coupling and laser-induced cavitation using embedded boundary and level set methods.
Journal of Computational Physics,
Vol. 472,
Issue. ,
p.
111656.
Ma, Wentao
Zhao, Xuning
Islam, Shafquat
Narkhede, Aditya
and
Wang, Kevin
2023.
Efficient solution of bimaterial Riemann problems for compressible multi-material flow simulations.
Journal of Computational Physics,
Vol. 493,
Issue. ,
p.
112474.
Jiang, Xudong
Wang, Yuxiang
and
Sun, Cheng
2023.
Numerical investigation of the dynamic response of a simplified floating structure subjected to two underwater explosion bubbles.
Journal of Mechanical Science and Technology,
Vol. 37,
Issue. 11,
p.
5623.
Dular, Matevž
and
Ohl, Claus Dieter
2023.
Bulk material influence on the aggressiveness of cavitation – Questioning the microjet impact influence and suggesting a possible way to erosion mitigation.
Wear,
Vol. 530-531,
Issue. ,
p.
205061.
Zhao, Ben
and
Coutier-Delgosha, Olivier
2023.
The impacts of material acoustic impedance and thickness on single laser-induced bubble dynamics and determining factors in resulting pressure.
Physics of Fluids,
Vol. 35,
Issue. 10,
Islam, Shafquat T.
Ma, Wentao
Michopoulos, John G.
and
Wang, Kevin
2023.
Plasma formation in ambient fluid from hypervelocity impacts.
Extreme Mechanics Letters,
Vol. 58,
Issue. ,
p.
101927.
Xu, Zeyang
Chai, Yuao
Ma, Liqiang
and
Zhu, Yiding
2024.
The visualization of an ultrasonic standing wave field using scanning-focused laser differential interferometry.
Physics of Fluids,
Vol. 36,
Issue. 7,
Li, Jie
Luo, Jing
Xu, Weilin
Zhai, Yanwei
Bai, Lixin
Qu, Tong
and
Fu, Guihua
2024.
Experimental study on effect of elastic-rigid composite boundary on shockwave from cavitation bubble collapse.
Physics of Fluids,
Vol. 36,
Issue. 11,
Shams, Armand
Bidi, Saeed
and
Gavaises, Manolis
2024.
Investigation of the ultrasound-induced collapse of air bubbles near soft materials.
Ultrasonics Sonochemistry,
Vol. 102,
Issue. ,
p.
106723.
Zhao, Xuning
Ma, Wentao
Chen, Junqin
Xiang, Gaoming
Zhong, Pei
and
Wang, Kevin
2024.
Vapour bubbles produced by long-pulsed laser: a race between advection and phase transition.
Journal of Fluid Mechanics,
Vol. 999,
Issue. ,
Abbondanza, Dario
Gallo, Mirko
and
Casciola, Carlo Massimo
2024.
Collapse of microbubbles over an elastoplastic wall.
Journal of Fluid Mechanics,
Vol. 999,
Issue. ,
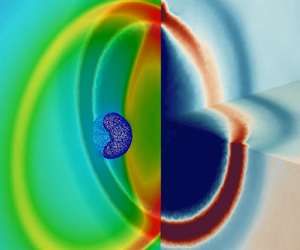
 $Z/Z_0$, plays a significant role. When
$Z/Z_0$, plays a significant role. When  $Z/Z_0<1$, the material reflects the compressive front of the incident shock into a tensile wave. The reflected tensile wave impinges on the bubble and decelerates its collapse. As a result, the collapse produces a liquid jet, but not necessarily a shock wave. When
$Z/Z_0<1$, the material reflects the compressive front of the incident shock into a tensile wave. The reflected tensile wave impinges on the bubble and decelerates its collapse. As a result, the collapse produces a liquid jet, but not necessarily a shock wave. When  $Z/Z_0>1$, the reflected wave is compressive and accelerates the bubble's collapse, leading to the emission of a shock wave whose amplitude increases linearly with
$Z/Z_0>1$, the reflected wave is compressive and accelerates the bubble's collapse, leading to the emission of a shock wave whose amplitude increases linearly with  $\log (Z/Z_0)$, and can be much higher than the amplitude of the incident shock. The reflection of this emitted shock wave impinges on the bubble during its rebound. It reduces the speed of the bubble's rebound and the velocity of the liquid jet. Furthermore, we show that, for a set of materials with
$\log (Z/Z_0)$, and can be much higher than the amplitude of the incident shock. The reflection of this emitted shock wave impinges on the bubble during its rebound. It reduces the speed of the bubble's rebound and the velocity of the liquid jet. Furthermore, we show that, for a set of materials with  $Z/Z_0\in [0.04, 10.8]$, the effect of acoustic impedance on the bubble's collapse time and minimum volume can be captured using phenomenological models constructed based on the solution of Rayleigh–Plesset equation.
$Z/Z_0\in [0.04, 10.8]$, the effect of acoustic impedance on the bubble's collapse time and minimum volume can be captured using phenomenological models constructed based on the solution of Rayleigh–Plesset equation.