Article contents
Shock interactions in two-dimensional steady flows of Bethe–Zel’dovich–Thompson fluids
Published online by Cambridge University Press: 23 January 2020
Abstract
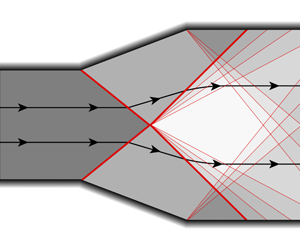
The morphology of nodes generated by the interaction of discontinuities in steady two-dimensional inviscid flows is examined. The fluids considered are Bethe–Zel’dovich–Thompson (BZT) fluids, which feature negative values of the fundamental derivative of gas dynamics in the vapour phase. The operating conditions correspond to the non-classical gas-dynamic regime where expansion shocks, compression fans and composite waves are admissible in addition to the classical compression shocks and expansion fans. Interactions caused by the crossing, overtaking and splitting of compression/expansion shocks, along with the refraction of these through a contact discontinuity, are analysed here. The well-established method of wave curves is applied to non-classical wave curves, revealing a variety of interaction patterns that are simply not admissible in classical gas dynamics. It is shown that shock waves can be reflected, transmitted and refracted as Prandtl–Meyer fans or composite waves. Based on numerical evidence, the splitting (and consequently the Mach reflection) of an expansion shock seems to be disallowed. Theoretical considerations on the admissibility of such configurations are also provided. The present analysis is relevant to applications potentially involving supersonic flows of BZT fluids, e.g. organic Rankine cycle power systems, and can also be used in front-tracking algorithms for general equations of state.
JFM classification
Information
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2020. Published by Cambridge University Press
References
- 4
- Cited by

