No CrossRef data available.
Article contents
A scaling law for the lift of a bio-inspired wing hovering in low-density compressible flows
Published online by Cambridge University Press: 13 January 2025
Abstract
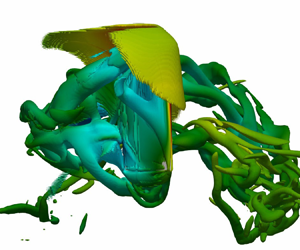
Aircraft with bio-inspired flapping wings that are operated in low-density atmospheric environments encounter unique challenges associated with the low density. The low density results in the requirement of high operating velocities of aircraft to generate sufficient lift resulting in significant compressibility effects. Here, we perform numerical simulations to investigate the compressibility effects on the lift generation of a bio-inspired wing during hovering flight using an immersed boundary method. The aim of this study is to develop a scaling law to understand how the lift is influenced by the Reynolds and Mach numbers, and the associated flow physics. Our simulations have identified a critical Mach number of approximately  $0.6$ defined by the average wing-tip velocity. When the Mach number is lower than 0.6, compressibility does not have significant effects on the lift or flow fields, while when the Mach number is greater than
$0.6$ defined by the average wing-tip velocity. When the Mach number is lower than 0.6, compressibility does not have significant effects on the lift or flow fields, while when the Mach number is greater than  $0.6$, the lift coefficient decreases linearly with increasing Mach number, due to the drastic change in the pressure on the wing surface caused by unsteady shock waves. Moreover, the decay rate is dependent on the Reynolds number and the angle of attack. Based on these observations, we propose a scaling law for the lift of a hovering flapping wing by considering compressible and viscous effects, with the scaled lift showing excellent collapse.
$0.6$, the lift coefficient decreases linearly with increasing Mach number, due to the drastic change in the pressure on the wing surface caused by unsteady shock waves. Moreover, the decay rate is dependent on the Reynolds number and the angle of attack. Based on these observations, we propose a scaling law for the lift of a hovering flapping wing by considering compressible and viscous effects, with the scaled lift showing excellent collapse.
JFM classification
Information
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2025. Published by Cambridge University Press


