Hostname: page-component-745bb68f8f-lrblm Total loading time: 0 Render date: 2025-01-26T21:54:41.778Z Has data issue: false hasContentIssue false
  • English
  • Français

Rotating horizontal convection

Published online by Cambridge University Press:  16 April 2013

Roy Barkan ,
Kraig B. Winters  and
Stefan G. Llewellyn Smith
Roy Barkan*
Affiliation:
Scripps Institution of Oceanography, UCSD, 9500 Gilman Drive, La Jolla CA 92093-0209, USA
Kraig B. Winters
Affiliation:
Scripps Institution of Oceanography, UCSD, 9500 Gilman Drive, La Jolla CA 92093-0209, USA Department of Mechanical and Aerospace Engineering, Jacobs School of Engineering, UCSD, 9500 Gilman Drive, La Jolla CA 92093-0411, USA
Stefan G. Llewellyn Smith
Affiliation:
Department of Mechanical and Aerospace Engineering, Jacobs School of Engineering, UCSD, 9500 Gilman Drive, La Jolla CA 92093-0411, USA
*
†Email address for correspondence: rbarkan@ucsd.edu
Get access Rights & Permissions [Opens in a new window]

Abstract

‘Horizontal convection’ (HC) is the generic name for the flow resulting from a buoyancy variation imposed along a horizontal boundary of a fluid. We study the effects of rotation on three-dimensional HC numerically in two stages: first, when baroclinic instability is suppressed and, second, when it ensues and baroclinic eddies are formed. We concentrate on changes to the thickness of the near-surface boundary layer, the stratification at depth, the overturning circulation and the flow energetics during each of these stages. Our results show that, for moderate flux Rayleigh numbers (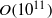$O(1{0}^{11} )$), rapid rotation greatly alters the steady-state solution of HC. When the flow is constrained to be uniform in the transverse direction, rapidly rotating solutions do not support a boundary layer, exhibit weaker overturning circulation and greater stratification at all depths. In this case, diffusion is the dominant mechanism for lateral buoyancy flux and the consequent buildup of available potential energy leads to baroclinically unstable solutions. When these rapidly rotating flows are perturbed, baroclinic instability develops and baroclinic eddies dominate both the lateral and vertical buoyancy fluxes. The resulting statistically steady solution supports a boundary layer, larger values of deep stratification and multiple overturning cells compared with non-rotating HC. A transformed Eulerian-mean approach shows that the residual circulation is dominated by the quasi-geostrophic eddy streamfunction and that the eddy buoyancy flux has a non-negligible interior diabatic component. The kinetic and available potential energies are greater than in the non-rotating case and the mixing efficiency drops from 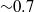${\sim }0. 7$ to 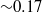${\sim }0. 17$. The eddies play an important role in the formation of the thermal boundary layer and, together with the negatively buoyant plume, help establish deep stratification. These baroclinically active solutions have characteristics of geostrophic turbulence.

JFM classification

Convection: Convection Geophysical and Geological Flows: Geostrophic turbulence Geophysical and Geological Flows: Ocean processes
Type
Papers
Information
Journal of Fluid Mechanics , Volume 723 , 25 May 2013 , pp. 556 - 586
Copyright
©2013 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Andrews, D. G 1981 A note on potential energy density in a stratified incompressible fluid. J. Fluid Mech. 107, 227–236.CrossRefGoogle Scholar
Andrews, D. G. & McIntyre, M. E. 1976 Planetary waves in horizontal and vertical shear: the generalized Eliassen–Palm relation and the mean zonal acceleration. J. Atmos. Sci. 33, 2031–2048.Google Scholar
Andrews, D. G. & McIntyre, M. E. 1978 Generalized Eliassen–Palm and Charney–Drazin theorems for waves on axisymmetric mean flows in compressible atmospheres. J. Atmos. Sci. 35, 175–185.Google Scholar
Beardsley, R. C. & Festa, J. F. 1972 A numerical model of convection driven by a surface stress and non-uniform horizontal heating. J. Phys. Oceanogr. 2 (4), 444–455.2.0.CO;2>CrossRefGoogle Scholar
Bryan, K. & Cox, M. D. 1967 A numerical investigation of oceanic general circulation. Tellus 19, 54–80.Google Scholar
Cessi, P. & Fantini, M. 2004 The eddy-driven thermocline. J. Phys. Oceanogr. 34, 2642–2658.Google Scholar
Chiu-Webster, S., Hinch, E. J. & Lister, J. 2008 Very viscous horizontal convection. J. Fluid Mech. 611, 395–426.CrossRefGoogle Scholar
Coman, M. A., Griffiths, R. W. & Hughes, G. O. 2006 Sandström’s experiments revisited. J. Mar. Res. 64, 783–796.Google Scholar
Eady, E. 1949 Long waves and cyclone waves. Tellus 1, 33–52.CrossRefGoogle Scholar
Hazewinkel, J., Paparella, F. & Young, W. R. 2012 Stressed horizontal convection. J. Fluid Mech. 692, 317–331.CrossRefGoogle Scholar
Henning, C. & Vallis, G. K. 2004 The effects of mesoscale eddies on the main subtropical thermocline. J. Phys. Oceanogr. 34, 2428–2443.Google Scholar
Hignett, P., Ibbetson, A. & Killworth, P. D. 1981 On rotating thermal convection driven by non-uniform heating from below. J. Fluid Mech. 109, 161–187.Google Scholar
Holliday, D. & McIntyre, ME 1981 On potential energy density in an incompressible stratified fluid. J. Fluid Mech. 107, 221–225.Google Scholar
Hughes, G. O. & Griffiths, R. W. 2008 Horizontal convection. Annu. Rev. Fluid Mech. 40, 185–208.CrossRefGoogle Scholar
Hughes, G. O., Hogg, A. M. & Griffiths, R. W. 2009 Available potential energy and irreversible mixing in the meridional overturning circulation. J. Phys. Oceanogr. 39, 3130–3146.Google Scholar
Ilicak, M. & Vallis, G. K. 2012 Simulations and scaling of horizontal convection. Tellus A 64, 18377.Google Scholar
Jeffreys, H. 1925 On fluid motions produced by differences of temperature and humidity. Q. J. R. Meteorol. Soc. 51, 347–356.Google Scholar
Killworth, P. D. & Manins, P. C. 1980 A model of confined thermal convection driven by non-uniform heating from below. J. Fluid Mech. 98, 587–607.CrossRefGoogle Scholar
King, E. M., Stellmach, S., Noir, J., Hansen, U. & Aurnou, J. M. 2009 Boundary layer control of rotating convection systems. Nature 457, 301–304.Google Scholar
Marshall, J., Jones, H., Karsten, R. & Wardle, R. 2002 Can eddies set ocean stratification? J. Phys. Oceanogr. 32, 26–38.2.0.CO;2>CrossRefGoogle Scholar
Marshall, J. & Radko, T. 2003 Residual-mean solutions for the antarctic circumpolar current and its associated overturning circulation. J. Phys. Oceanogr. 33, 2341–2354.Google Scholar
Marshall, J & Schott, F. 1999 Open-ocean convection: observations, theory and models. Rev. Geophys. 37, 1–64.Google Scholar
Molemaker, M. J. & McWilliams, J. C. 2010 Local balance and cross-scale flux of available potential energy. J. Fluid Mech. 645, 295–314.Google Scholar
Mullarney, J. C., Griffiths, R. W. & Hughes, G. O. 2004 Convection driven by differential heating at a horizontal boundary. J. Fluid Mech. 516, 181–209.Google Scholar
Munk, W. H. & Wunsch, C. 1998 Abyssal recipes II: energetics of tidal and wind mixing. Deep-Sea Res. 45, 1977–2010.Google Scholar
Paparella, F. & Young, W. R. 2002 Horizontal convection is non-turbulent. J. Fluid Mech. 466, 205–14.Google Scholar
Park, Y. G. & Whitehead, J. A. 1999 Rotating convection driven by differential bottom heating. J. Phys. Oceanogr. 29, 1208–1220.Google Scholar
Peltier, W. & Caulfield, C. 2003 Mixing efficiency in stratified shear flows. Annu. Rev. Fluid Mech. 35, 135–167.Google Scholar
Perez-Perez, E., Read, P. L. & Moroz, I. M. 2010 Assessing eddy parameterization schemes in a differentially heated rotating annulus experiment. Ocean Model. 32, 118–131.Google Scholar
Plumb, R. A. & Ferrari, R. 2005 Transformed Eulerian-mean theory. Part I: nonquasigeostrophic theory for eddies on a zonal-mean flow. J. Phys. Oceanogr. 35, 165–174.Google Scholar
Robinson, A. & Stommel, H. 1959 The oceanic thermocline and the associated thermohaline circulation. Tellus 11, 295–308.CrossRefGoogle Scholar
Rossby, T. 1965 On thermal convection driven by non-uniform heating from below: an experimental study. Deep-Sea Res. 12, 9–16.Google Scholar
Rossby, T. 1998 Numerical experiments with a fluid heated non-uniformly from below. Tellus 50A, 242–257.Google Scholar
Roullet, G. & Klein, P. 2009 Available potential energy diagnosis in a direct numerical simulation of rotating stratified turbulence. J. Fluid Mech. 624, 45–55.CrossRefGoogle Scholar
Salmon, R. 1980 Baroclinic instability and geostrophic turbulence. Geophys. Astrophys. Fluid Dyn. 15, 165–211.Google Scholar
Sandström, J. W. 1908 Dynamishce versuche mit merrwasser. Ann. Hydrogr. Marit. Meteorol. 36, 6–23.Google Scholar
Sandström, J. W. 1916 Meteorologische studien im Schwedischen Hochgebirge. Göteb. Kungl. Vetensk. Vitterh. Handl. 17, 1–48.Google Scholar
Scotti, A., Beardsley, R. & Butman, B. 2006 On the interpretation of energy and energy fluxes of nonlinear internal waves: an example from Massachusetts Bay. J. Fluid Mech. 561, 103–112.CrossRefGoogle Scholar
Scotti, A. & White, B. 2011 Is horizontal convection really non-turbulent? Geophys. Res. Lett. 38, L21609.CrossRefGoogle Scholar
Smith, K. S., Boccaletti, G., Hennings, C. C., Marinov, I., Tam, C. Y., Held, I. M. & Vallis, G. K. 2002 Turbulent diffusion in the geostrophic inverse cascade. J. Fluid Mech. 469, 13–48.CrossRefGoogle Scholar
Smith, R. 1976 Longitudinal dispersion of a buoyant contaminant in a shallow channel. J. Fluid Mech. 78, 677–688.CrossRefGoogle Scholar
Stern, M. E. 1975 Ocean Circulation Physics. Academic.Google Scholar
Stewart, K. D. 2012 The effect of sills and mixing on the meridional overturning circulation. PhD thesis, Australian National University, 128 pp.CrossRefGoogle Scholar
Stone, P. H. 1966 On non-geostrophic baroclinic instability. J. Atmos. Sci. 23, 390–400.Google Scholar
Tailleux, R. 2009 On the energetics of turbulent mixing, irreversible thermodynamics, Boussinesq models, and the ocean heat engine controversy. J. Fluid Mech. 638, 339–382.Google Scholar
Tailleux, R. & Rouleau, L. 2010 The effect of mechanical stirring on horizontal convecion. Tellus 62A, 138–153.Google Scholar
Vallis, G. K. 2006 Atmospheric and Oceanic Fluid Dynamics. Cambridge University Press.Google Scholar
Welander, P. 1971 The thermocline problem. Phil. Trans. R. Soc. Lond. A 270, 415–421.Google Scholar
Winters, K. B., Lombard, P. N., Riley, J. J. & D’Asaro, E. A. 1995 Available potential energy and mixing in density stratified fluids. J. Fluid Mech. 289, 115–128.Google Scholar
Winters, K. B. & Young, W. R. 2009 Available potential energy and buoyancy variance in horizontal convection. J. Fluid Mech. 629, 221–230.Google Scholar
Winters, K. B. & de la Fuente, A. 2012 Modelling rotating stratified flows at laboratory-scale using spectrally-based DNS. J. Oceangr. Mod. 49–50, 47–59.Google Scholar
Winters, K. B. & Barkan, R. 2013 Available potential energy density for Boussinesq fluid flow. J. Fluid Mech. 714, 476–488.Google Scholar
Whitehead, J. A. 1981 Laboratory models of circulation in shallow seas. Phil. Trans. R. Soc. Lond. A 302, 583–595.Google Scholar
Whitehead, J. A. & Wang, w. 2008 A laboratory model of vertical ocean circulation driven by mixing. J. Phys. Oceanogr. 38, 1091–1106.CrossRefGoogle Scholar
Wolfe, C. L. 2013 Approximations to the ocean’s residual overturning circulation. J. Oceangr. Mod. (submitted).Google Scholar
Wolfe, C. L. & Cessi, P. 2010 What sets the strength of the mid-depth stratification and overturning circulation in eddying ocean models? J. Phys. Oceanogr. 40, 1520–1538.Google Scholar
Wunsch, C. & Ferrari, R. 2004 Vertical mixing, energy, and the general circulation of the oceans. Annu. Rev. Fluid Mech. 36, 281–314.Google Scholar