Article contents
Pulsed jets driven by two interacting cavitation bubbles produced at different times
Published online by Cambridge University Press: 27 April 2017
Abstract
An experiment is performed using high-speed photography to elucidate the behaviours of jets formed by the interactions of two laser-induced tandem bubbles produced axisymmetrically for a range of dimensionless interaction parameters such as the bubble size ratio, 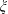 $\unicode[STIX]{x1D709}$ , the distance between the two cavitation bubbles,
$\unicode[STIX]{x1D709}$ , the distance between the two cavitation bubbles, 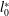 $l_{0}^{\ast }$ , and the time difference in bubble generation,
$l_{0}^{\ast }$ , and the time difference in bubble generation, 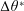 $\unicode[STIX]{x0394}\unicode[STIX]{x1D703}^{\ast }$ . A strong interaction occurs for
$\unicode[STIX]{x0394}\unicode[STIX]{x1D703}^{\ast }$ . A strong interaction occurs for 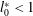 $l_{0}^{\ast }<1$ . The first bubble produced (bubble A) deforms because of the rapid growth of the second bubble (bubble B) to create a pulsed conical jet, sometimes with spray formation at the tip, formed by the small amount of water confined between the two bubbles. This phenomenon is followed by bubble penetration, toroidal bubble collapse, and the subsequent fast contraction of bubble B accompanied by a fine jet. The formation mechanism of the conical jet is similar to that of a water spike developed in air from a deformed free surface of a single growing bubble; however, the pressures of the gases surrounding each type of jet differ. The jet behaviours can be controlled by manipulating the interaction parameters; the jet velocity is significantly affected by
$l_{0}^{\ast }<1$ . The first bubble produced (bubble A) deforms because of the rapid growth of the second bubble (bubble B) to create a pulsed conical jet, sometimes with spray formation at the tip, formed by the small amount of water confined between the two bubbles. This phenomenon is followed by bubble penetration, toroidal bubble collapse, and the subsequent fast contraction of bubble B accompanied by a fine jet. The formation mechanism of the conical jet is similar to that of a water spike developed in air from a deformed free surface of a single growing bubble; however, the pressures of the gases surrounding each type of jet differ. The jet behaviours can be controlled by manipulating the interaction parameters; the jet velocity is significantly affected by 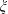 $\unicode[STIX]{x1D709}$ and
$\unicode[STIX]{x1D709}$ and 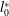 $l_{0}^{\ast }$ , but less so by
$l_{0}^{\ast }$ , but less so by  $\unicode[STIX]{x0394}\unicode[STIX]{x1D703}^{\ast }$ for
$\unicode[STIX]{x0394}\unicode[STIX]{x1D703}^{\ast }$ for  $\unicode[STIX]{x0394}\unicode[STIX]{x1D703}^{\ast }>\unicode[STIX]{x0394}\unicode[STIX]{x1D703}_{c}^{\ast }$ (
$\unicode[STIX]{x0394}\unicode[STIX]{x1D703}^{\ast }>\unicode[STIX]{x0394}\unicode[STIX]{x1D703}_{c}^{\ast }$ ( 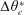 $\unicode[STIX]{x0394}\unicode[STIX]{x1D703}_{c}^{\ast }$ being the critical birth-time difference). The optimum time of jet impact, at which bubble A reaches its maximum volume, depends on
$\unicode[STIX]{x0394}\unicode[STIX]{x1D703}_{c}^{\ast }$ being the critical birth-time difference). The optimum time of jet impact, at which bubble A reaches its maximum volume, depends on 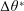 $\unicode[STIX]{x0394}\unicode[STIX]{x1D703}^{\ast }$ . It is generally later for larger values of
$\unicode[STIX]{x0394}\unicode[STIX]{x1D703}^{\ast }$ . It is generally later for larger values of 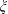 $\unicode[STIX]{x1D709}$ . A pulsed jet could be used to create small pores in a cell membrane; therefore, the reported method may be useful for application in tandem-bubble sonoporation.
$\unicode[STIX]{x1D709}$ . A pulsed jet could be used to create small pores in a cell membrane; therefore, the reported method may be useful for application in tandem-bubble sonoporation.
JFM classification
Information
- Type
- Papers
- Information
- Copyright
- © 2017 Cambridge University Press
References
- 47
- Cited by


