Published online by Cambridge University Press: 02 December 2021
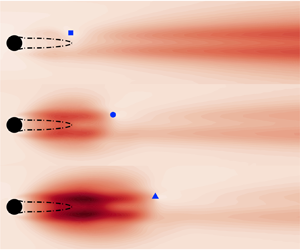
We consider linear feedback control of the two-dimensional flow past a cylinder at low Reynolds numbers, with a particular focus on the optimal placement of a single sensor and a single actuator. To accommodate the high dimensionality of the flow, we compute its leading resolvent forcing and response modes to enable the design of  $\mathcal {H}_2$-optimal estimators and controllers. We then investigate three control problems: (i) optimal estimation (OE) in which we measure the flow at a single location and estimate the entire flow; (ii) full-state information control (FIC) in which we measure the entire flow but actuate at only one location; and (iii) the overall feedback control problem in which a single sensor is available for measurement and a single actuator is available for control. We characterize the performance of these control arrangements over a range of sensor and actuator placements and discuss implications for effective feedback control when using a single sensor and a single actuator. The optimal sensor and actuator placements found for the OE and FIC problems are also compared with those found for the overall feedback control problem over a range of Reynolds numbers. This comparison reveals the key factors and conflicting trade-offs that limit feedback control performance.
$\mathcal {H}_2$-optimal estimators and controllers. We then investigate three control problems: (i) optimal estimation (OE) in which we measure the flow at a single location and estimate the entire flow; (ii) full-state information control (FIC) in which we measure the entire flow but actuate at only one location; and (iii) the overall feedback control problem in which a single sensor is available for measurement and a single actuator is available for control. We characterize the performance of these control arrangements over a range of sensor and actuator placements and discuss implications for effective feedback control when using a single sensor and a single actuator. The optimal sensor and actuator placements found for the OE and FIC problems are also compared with those found for the overall feedback control problem over a range of Reynolds numbers. This comparison reveals the key factors and conflicting trade-offs that limit feedback control performance.