Article contents
On the rheology and magnetization of dilute magnetic emulsions under small amplitude oscillatory shear
Published online by Cambridge University Press: 12 January 2023
Abstract
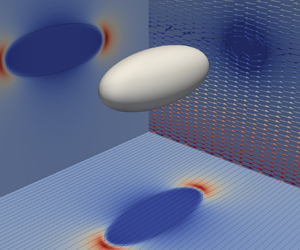
A dilute magnetic emulsion under the combined action of a uniform external magnetic field and a small amplitude oscillatory shear is studied using numerical simulations. We consider a three-dimensional domain with a single ferrofluid droplet suspended in a non-magnetizable Newtonian fluid. We present results of droplet shape and orientation, viscoelastic functions and bulk emulsion magnetization as functions of the shear oscillation frequency, magnetic field intensity and orientation. We also investigate how the magnetic field induces mechanical anisotropy by producing internal torques in oscillatory conditions. We found that, when the magnetic field is parallel to the shear plane, the droplet shape is mostly independent of the shear oscillation frequency. Regarding the viscometric functions, we show how the external magnetic field modifies the storage and loss moduli, especially for a field aligned to the main velocity gradient. The bulk emulsion magnetization is studied in the same fashion as the viscoelastic functions of the oscillatory shear. We show that the in-phase component of the magnetization with respect to the shear rate reaches a saturation magnetization, at the high frequencies limit, dependent on the magnetic field intensity and orientation. On the other hand, we found a non-zero out-of-phase response, which indicates a finite emulsion magnetization relaxation time. Our results indicate that the magnetization relaxation is closely related to the mechanical relaxation for dilute magnetic emulsions under oscillatory shear.
JFM classification
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2023. Published by Cambridge University Press
References
REFERENCES
- 3
- Cited by



