Published online by Cambridge University Press: 01 October 2012
In the present study, shock tube experiments are used to study the very late-time development of the Richtmyer–Meshkov instability from a diffuse, nearly sinusoidal, initial perturbation into a fully turbulent flow. The interface is generated by two opposing gas flows and a perturbation is formed on the interface by transversely oscillating the shock tube to create a standing wave. The puncturing of a diaphragm generates a Mach 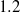 $1. 2$ shock wave that then impacts a density gradient composed of air and SF6, causing the Richtmyer–Meshkov instability to develop in the 2.0 m long test section. The instability is visualized with planar Mie scattering in which smoke particles in the air are illuminated by a Nd:YLF laser sheet, and images are recorded using four high-speed video cameras operating at 6 kHz that allow the recording of the time history of the instability. In addition, particle image velocimetry (PIV) is implemented using a double-pulsed Nd:YAG laser with images recorded using a single CCD camera. Initial modal content, amplitude, and growth rates are reported from the Mie scattering experiments while vorticity and circulation measurements are made using PIV. Amplitude measurements show good early-time agreement but relatively poor late-time agreement with existing nonlinear models. The model of Goncharov (Phys. Rev. Lett., vol. 88, 2002, 134502) agrees with growth rate measurements at intermediate times but fails at late experimental times. Measured background acceleration present in the experiment suggests that the late-time growth rate may be influenced by Rayleigh–Taylor instability induced by the interfacial acceleration. Numerical simulations conducted using the LLNL codes Ares and Miranda show that this acceleration may be caused by the growth of boundary layers, and must be accounted for to produce good agreement with models and simulations. Adding acceleration to the Richtmyer–Meshkov buoyancy–drag model produces improved agreement. It is found that the growth rate and amplitude trends are also modelled well by the Likhachev–Jacobs vortex model (Likhachev & Jacobs, Phys. Fluids, vol. 17, 2005, 031704). Circulation measurements also show good agreement with the circulation value extracted by fitting the vortex model to the experimental data.
$1. 2$ shock wave that then impacts a density gradient composed of air and SF6, causing the Richtmyer–Meshkov instability to develop in the 2.0 m long test section. The instability is visualized with planar Mie scattering in which smoke particles in the air are illuminated by a Nd:YLF laser sheet, and images are recorded using four high-speed video cameras operating at 6 kHz that allow the recording of the time history of the instability. In addition, particle image velocimetry (PIV) is implemented using a double-pulsed Nd:YAG laser with images recorded using a single CCD camera. Initial modal content, amplitude, and growth rates are reported from the Mie scattering experiments while vorticity and circulation measurements are made using PIV. Amplitude measurements show good early-time agreement but relatively poor late-time agreement with existing nonlinear models. The model of Goncharov (Phys. Rev. Lett., vol. 88, 2002, 134502) agrees with growth rate measurements at intermediate times but fails at late experimental times. Measured background acceleration present in the experiment suggests that the late-time growth rate may be influenced by Rayleigh–Taylor instability induced by the interfacial acceleration. Numerical simulations conducted using the LLNL codes Ares and Miranda show that this acceleration may be caused by the growth of boundary layers, and must be accounted for to produce good agreement with models and simulations. Adding acceleration to the Richtmyer–Meshkov buoyancy–drag model produces improved agreement. It is found that the growth rate and amplitude trends are also modelled well by the Likhachev–Jacobs vortex model (Likhachev & Jacobs, Phys. Fluids, vol. 17, 2005, 031704). Circulation measurements also show good agreement with the circulation value extracted by fitting the vortex model to the experimental data.
Present address: Roxar Flow Measurement, Gamle Forusvei 17, 4065 Stavanger, Norway.
Present address: Lockheed Martin Aeronautics, 1011 Lockheed Way, Palmdale, CA 93599.