Article contents
On mixing enhancement by secondary baroclinic vorticity in a shock–bubble interaction
Published online by Cambridge University Press: 26 November 2021
Abstract
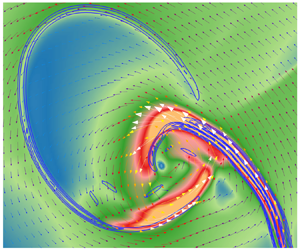
To investigate the intrinsic mechanism for mixing enhancement by variable-density (VD) behaviour, a canonical VD mixing extracted from a supersonic streamwise vortex protocol, a shock–bubble interaction (SBI), is numerically studied and compared with a counterpart of passive-scalar (PS) mixing. It is meaningful to observe that the maximum concentration decays much faster in a VD SBI than in a PS SBI regardless of the shock Mach number ( $Ma=1.22 - 4$). The quasi-Lamb–Oseen-type velocity distribution in the PS SBI is found by analysing the azimuthal velocity that stretches the bubble. Meanwhile, for the VD SBI, an additional stretching enhanced by the secondary baroclinic vorticity (SBV) production contributes to the faster-mixing decay. The underlying mechanism of the SBV-enhanced stretching is further revealed through the density and velocity difference between the light shocked bubble and the heavy ambient air. By combining the SBV-accelerated stretching model and the initial shock compression, a novel mixing time estimation for VD SBI is theoretically proposed by solving the advection–diffusion equation under a deformation field of an axisymmetric vortex with the additional SBV-induced azimuthal velocity. Based on the mixing time model, a mixing enhancement number, defined by the ratio of VD and PS mixing time further, reveals the contribution from the VD effect, which implies a better control of the density distribution for mixing enhancement in a supersonic streamwise vortex.
$Ma=1.22 - 4$). The quasi-Lamb–Oseen-type velocity distribution in the PS SBI is found by analysing the azimuthal velocity that stretches the bubble. Meanwhile, for the VD SBI, an additional stretching enhanced by the secondary baroclinic vorticity (SBV) production contributes to the faster-mixing decay. The underlying mechanism of the SBV-enhanced stretching is further revealed through the density and velocity difference between the light shocked bubble and the heavy ambient air. By combining the SBV-accelerated stretching model and the initial shock compression, a novel mixing time estimation for VD SBI is theoretically proposed by solving the advection–diffusion equation under a deformation field of an axisymmetric vortex with the additional SBV-induced azimuthal velocity. Based on the mixing time model, a mixing enhancement number, defined by the ratio of VD and PS mixing time further, reveals the contribution from the VD effect, which implies a better control of the density distribution for mixing enhancement in a supersonic streamwise vortex.
Information
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2021. Published by Cambridge University Press
References
REFERENCES
- 10
- Cited by


