Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Chen, Weilin
Ji, Chunning
Xu, Dong
and
Zhang, Zhimeng
2020.
Oscillation regimes and mechanisms in the vortex-induced vibrations of three circular cylinders with equilateral-triangular arrangements.
Physics of Fluids,
Vol. 32,
Issue. 4,
Yan, Yuhao
Ji, Chunning
and
Srinil, Narakorn
2020.
Three-dimensional flip-flopping flow around a pair of dual-stepped circular cylinders in a side-by-side arrangement.
Physics of Fluids,
Vol. 32,
Issue. 12,
Wang, Jia-song
Fan, Dixia
and
Lin, Ke
2020.
A review on flow-induced vibration of offshore circular cylinders.
Journal of Hydrodynamics,
Vol. 32,
Issue. 3,
p.
415.
Blanchard, Antoine B.
Cornejo Maceda, Guy Y.
Fan, Dewei
Li, Yiqing
Zhou, Yu
Noack, Bernd R.
and
Sapsis, Themistoklis P.
2021.
Bayesian optimization for active flow control.
Acta Mechanica Sinica,
Vol. 37,
Issue. 12,
p.
1786.
Liu, Bin
2021.
A Nitsche stabilized finite element method: Application for heat and mass transfer and fluid–structure interaction.
Computer Methods in Applied Mechanics and Engineering,
Vol. 386,
Issue. ,
p.
114101.
Freidooni, F.
Sohankar, A.
Rastan, M.R.
and
Shirani, E.
2021.
Flow field around two tandem non-identical-height square buildings via LES.
Building and Environment,
Vol. 201,
Issue. ,
p.
107985.
Cornejo Maceda, Guy Y.
Li, Yiqing
Lusseyran, François
Morzyński, Marek
and
Noack, Bernd R.
2021.
Stabilization of the fluidic pinball with gradient-enriched machine learning control.
Journal of Fluid Mechanics,
Vol. 917,
Issue. ,
Zhang, Zhimeng
Ji, Chunning
Chen, Weilin
Hua, Yang
and
Srinil, Narakorn
2021.
Influence of boundary layer thickness and gap ratios on three-dimensional flow characteristics around a circular cylinder in proximity to a bottom plane.
Ocean Engineering,
Vol. 226,
Issue. ,
p.
108858.
Yan, Yuhao
Ji, Chunning
and
Srinil, Narakorn
2021.
On wake modulation and interaction features of a pair of dual-step circular cylinders in side-by-side arrangements.
Physics of Fluids,
Vol. 33,
Issue. 9,
Deng, Nan
Noack, Bernd R.
Morzyński, Marek
and
Pastur, Luc R.
2021.
Galerkin force model for transient and post-transient dynamics of the fluidic pinball.
Journal of Fluid Mechanics,
Vol. 918,
Issue. ,
Bai, X.
Ji, C.
Grant, P.
Phillips, N.
Oza, U.
Avital, E. J.
and
Williams, J. J. R.
2021.
Turbulent flow simulation of a single-blade Magnus rotor.
Advances in Aerodynamics,
Vol. 3,
Issue. 1,
Deng, Nan
Pastur, Luc R.
Tuckerman, Laurette S.
and
Noack, Bernd R.
2021.
Coinciding local bifurcations in the Navier-Stokes equations.
EPL (Europhysics Letters),
Vol. 135,
Issue. 2,
p.
24002.
Zhu, Hongjun
Chu, Xin
Yan, Zhiyin
and
Gao, Yun
2021.
Coupling response of flow-induced oscillating cylinder with a pair of flow-induced rotating impellers.
Physics of Fluids,
Vol. 33,
Issue. 8,
Voskoboinick, Volodymyr
Onyshchenko, Arthur
Voskoboinyk, Oleksandr
Makarenkova, Anastasiia
and
Voskobiinyk, Andrij
2022.
Junction flow inside and around three-row cylindrical group on rigid flat surface.
Heliyon,
Vol. 8,
Issue. 12,
p.
e12595.
Li, Ang
Shi, Shengmin
and
Fan, Dixia
2022.
Fluid Forces and Vortex Patterns of an Oscillating Cylinder Pair in Still Water With Both Fixed Side-by-Side and Tandem Configurations.
Journal of Offshore Mechanics and Arctic Engineering,
Vol. 144,
Issue. 2,
Chen, Weilin
Ji, Chunning
Srinil, Narakorn
Yan, Yuhao
and
Zhang, Zhimeng
2022.
Effects of upstream wake on vortex-induced vibrations and wake patterns of side-by-side circular cylinders.
Marine Structures,
Vol. 84,
Issue. ,
p.
103223.
Chen, Weilin
Ji, Chunning
Alam, Md. Mahbub
and
Yan, Yuhao
2022.
Three-dimensional flow past two stationary side-by-side circular cylinders.
Ocean Engineering,
Vol. 244,
Issue. ,
p.
110379.
Voskoboinick, Volodymyr
Onyshchenko, Arthur
Voskoboinyk, Oleksandr
Makarenkova, Anastasiia
and
Voskobiinyk, Andrij
2022.
Junction Flow About Cylindrical Group on Rigid Flat Surface.
SSRN Electronic Journal ,
Wang, Yisen
Yang, Zhonghua
Yu, Minghui
Zhou, Haiyan
and
Zhang, Dawei
2022.
Study on flow characteristics and diversity index of diamond-type boulder cluster with different spacing ratios.
Environmental Science and Pollution Research,
Vol. 29,
Issue. 23,
p.
34248.
Li, Songqi
Li, Wenpeng
and
Noack, Bernd R.
2022.
Machine-learned control-oriented flow estimation for multi-actuator multi-sensor systems exemplified for the fluidic pinball.
Journal of Fluid Mechanics,
Vol. 952,
Issue. ,
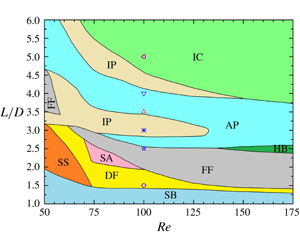
 $L/D(=1.0{-}6.0)$, Reynolds number
$L/D(=1.0{-}6.0)$, Reynolds number  $Re(=50{-}300)$ and three-dimensionality on the flow structures, hydrodynamic forces and Strouhal numbers, where
$Re(=50{-}300)$ and three-dimensionality on the flow structures, hydrodynamic forces and Strouhal numbers, where  $L$ is the cylinder centre-to-centre spacing and
$L$ is the cylinder centre-to-centre spacing and  $D$ is the cylinder diameter. The fluid dynamics involved is highly sensitive to both
$D$ is the cylinder diameter. The fluid dynamics involved is highly sensitive to both  $Re$ and
$Re$ and  $L/D$, leading to nine distinct flow structures, namely single bluff-body flow, deflected flow, flip-flopping flow, steady symmetric flow, steady asymmetric flow, hybrid flow, anti-phase flow, in-phase flow and fully developed in-phase co-shedding flow. The time-mean drag and lift of each cylinder are more sensitive to
$L/D$, leading to nine distinct flow structures, namely single bluff-body flow, deflected flow, flip-flopping flow, steady symmetric flow, steady asymmetric flow, hybrid flow, anti-phase flow, in-phase flow and fully developed in-phase co-shedding flow. The time-mean drag and lift of each cylinder are more sensitive to  $L/D$ than
$L/D$ than  $Re$ while fluctuating forces are less sensitive to
$Re$ while fluctuating forces are less sensitive to  $L/D$ than
$L/D$ than  $Re$. The three-dimensionality of the flow affects the development of the wake patterns, changing the
$Re$. The three-dimensionality of the flow affects the development of the wake patterns, changing the  $L/D$ ranges of different flow structures. A diagram of flow regimes, together with the contours of hydrodynamic forces, in the
$L/D$ ranges of different flow structures. A diagram of flow regimes, together with the contours of hydrodynamic forces, in the  $Re-L/D$ space, is given, providing physical insights into the complex interactions of the three cylinders.
$Re-L/D$ space, is given, providing physical insights into the complex interactions of the three cylinders.