Article contents
Normalized dissipation rate in a moderate Taylor Reynolds number flow
Published online by Cambridge University Press: 29 March 2017
Abstract
Time-resolved velocity measurements are obtained using a hot-wire in a nearly homogeneous and isotropic flow downstream of an active grid for a range of Taylor Reynolds numbers from 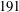 $191$ to
$191$ to 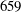 $659$ . It is found that the dimensionless dissipation rate,
$659$ . It is found that the dimensionless dissipation rate, 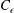 $C_{\unicode[STIX]{x1D716}}$ , is nearly a constant for sufficiently high values of Taylor Reynolds number,
$C_{\unicode[STIX]{x1D716}}$ , is nearly a constant for sufficiently high values of Taylor Reynolds number, 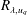 $R_{\unicode[STIX]{x1D706},u_{q}}$ , and is approximately equal to
$R_{\unicode[STIX]{x1D706},u_{q}}$ , and is approximately equal to 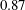 $0.87$ . This value is approximately
$0.87$ . This value is approximately 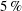 $5\,\%$ less than the value reported by Bos et al. (Phys. Fluids, vol. 19 (4), 2007, 045101), which is obtained using DNS/LES (direct numerical simulation combined with large eddy simulation) for decaying homogeneous and isotropic turbulence, and is in excellent agreement with the active grid experiment of Thormann & Meneveau (Phys. Fluids, vol. 26 (2), 2014, 025112.). The results presented herein show that deviation from isotropy may cause inconsistencies in the computation of
$5\,\%$ less than the value reported by Bos et al. (Phys. Fluids, vol. 19 (4), 2007, 045101), which is obtained using DNS/LES (direct numerical simulation combined with large eddy simulation) for decaying homogeneous and isotropic turbulence, and is in excellent agreement with the active grid experiment of Thormann & Meneveau (Phys. Fluids, vol. 26 (2), 2014, 025112.). The results presented herein show that deviation from isotropy may cause inconsistencies in the computation of 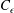 $C_{\unicode[STIX]{x1D716}}$ . As a result, it is suggested that the velocity scale be the square root of the turbulence kinetic energy. The integral length scale measurements obtained from the longitudinal velocity correlation are in close agreement with the integral length scale measured from the peak of the energy spectrum,
$C_{\unicode[STIX]{x1D716}}$ . As a result, it is suggested that the velocity scale be the square root of the turbulence kinetic energy. The integral length scale measurements obtained from the longitudinal velocity correlation are in close agreement with the integral length scale measured from the peak of the energy spectrum, 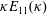 $\unicode[STIX]{x1D705}E_{11}(\unicode[STIX]{x1D705})$ , where
$\unicode[STIX]{x1D705}E_{11}(\unicode[STIX]{x1D705})$ , where  $\unicode[STIX]{x1D705}$ is the wavenumber and
$\unicode[STIX]{x1D705}$ is the wavenumber and 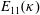 $E_{11}(\unicode[STIX]{x1D705})$ is the one-dimensional power spectrum of the downstream velocity.
$E_{11}(\unicode[STIX]{x1D705})$ is the one-dimensional power spectrum of the downstream velocity.
Information
- Type
- Papers
- Information
- Copyright
- © 2017 Cambridge University Press
References
- 14
- Cited by


