Article contents
Nonlinear flow-induced instability of an elastically mounted pitching wing
Published online by Cambridge University Press: 27 July 2020
Abstract
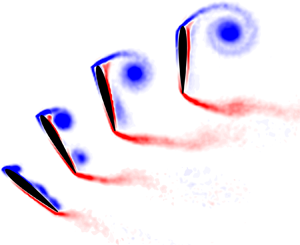
We experimentally study the nonlinear flow-induced instability of an elastically mounted pitching wing in a circulating water tunnel. The structural parameters of the finite-span wing are simulated and regulated using a cyber-physical control system. At a small fixed damping, we systematically vary the stiffness of the wing for different inertia values to test for the stability boundaries of the system. We observe that, for a high-inertia wing, the system dynamics bifurcates from stable fixed points to small-amplitude oscillations followed by large-amplitude limit-cycle oscillations (LCOs) via a subcritical bifurcation, which features hysteretic bistability and an abrupt amplitude jump. Under this condition, the pitching frequency of the wing locks onto its structural frequency and the oscillation is dominated by the inertial force, corresponding to a structural mode. Force and flow field measurements indicate the presence of a secondary leading-edge vortex (LEV). As the wing inertia decreases, the width of the bistable region shrinks. At a sufficiently low inertia, the pitching amplitude changes smoothly with the stiffness without any hysteresis, revealing a supercritical bifurcation. Under this condition, no lock-in phenomenon is observed and the pitching frequency remains relatively constant at a value lower than the structural frequency. Force decomposition shows dominating fluid force, indicating a hydrodynamic mode. The secondary LEV is absent. We show that the onset of large-amplitude LCOs in both the structural mode and the hydrodynamic mode scales with the Cauchy number, and the LCOs in the structural mode collapse with the non-dimensional velocity. We examine the subcritical transition in detail; we find that this transition depends on the static characteristics of the wing, and the secondary LEV starts to emerge at the early stage of the transition. Lastly, we adopt an energy approach to map out the stability of the system and explain the existence of the two distinct types of bifurcations observed for different inertia values.
JFM classification
Information
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2020. Published by Cambridge University Press
References
REFERENCES
- 22
- Cited by

