Article contents
Nonlinear exact coherent structures in pipe flow and their instabilities
Published online by Cambridge University Press: 15 April 2019
Abstract
In this paper, we present computational results of some two-fold azimuthally symmetric travelling waves and their stability. Calculations over a range of Reynolds numbers ( $Re$) reveal connections between a class of solutions computed by Wedin & Kerswell (J. Fluid Mech., vol. 508, 2004, pp. 333–371) (henceforth called the WK solution) and the
$Re$) reveal connections between a class of solutions computed by Wedin & Kerswell (J. Fluid Mech., vol. 508, 2004, pp. 333–371) (henceforth called the WK solution) and the 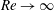 $Re\rightarrow \infty$ vortex–wave interaction theory of Hall & Smith (J. Fluid Mech., vol. 227, 1991, pp. 641–666) and Hall & Sherwin (J. Fluid Mech., vol. 661, 2010, pp. 178–205). In particular, the continuation of the WK solutions to larger values of
$Re\rightarrow \infty$ vortex–wave interaction theory of Hall & Smith (J. Fluid Mech., vol. 227, 1991, pp. 641–666) and Hall & Sherwin (J. Fluid Mech., vol. 661, 2010, pp. 178–205). In particular, the continuation of the WK solutions to larger values of 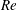 $Re$ shows that the WK solution bifurcates from a shift-and-rotate symmetric solution, which we call the WK2 state. The WK2 solution computed for
$Re$ shows that the WK solution bifurcates from a shift-and-rotate symmetric solution, which we call the WK2 state. The WK2 solution computed for 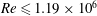 $Re\leqslant 1.19\times 10^{6}$ shows excellent agreement with the theoretical
$Re\leqslant 1.19\times 10^{6}$ shows excellent agreement with the theoretical  $Re^{-5/6}$,
$Re^{-5/6}$,  $Re^{-1}$ and
$Re^{-1}$ and 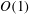 $O(1)$ scalings of the waves, rolls and streaks respectively. Furthermore, these states are found to have only two unstable modes in the large
$O(1)$ scalings of the waves, rolls and streaks respectively. Furthermore, these states are found to have only two unstable modes in the large 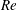 $Re$ regime, with growth rates estimated to be
$Re$ regime, with growth rates estimated to be 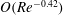 $O(Re^{-0.42})$ and
$O(Re^{-0.42})$ and  $O(Re^{-0.92})$, close to the theoretical
$O(Re^{-0.92})$, close to the theoretical 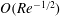 $O(Re^{-1/2})$ and
$O(Re^{-1/2})$ and 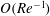 $O(Re^{-1})$ asymptotic results for edge and sinuous instability modes of vortex–wave interaction states (Deguchi & Hall, J. Fluid Mech., vol. 802, 2016, pp. 634–666) in plane Couette flow. For the nonlinear viscous core states (Ozcakir et al., J. Fluid Mech., vol. 791, 2016, pp. 284–328), characterized by spatial a shrinking of the wave and roll structure towards the pipe centre with increasing
$O(Re^{-1})$ asymptotic results for edge and sinuous instability modes of vortex–wave interaction states (Deguchi & Hall, J. Fluid Mech., vol. 802, 2016, pp. 634–666) in plane Couette flow. For the nonlinear viscous core states (Ozcakir et al., J. Fluid Mech., vol. 791, 2016, pp. 284–328), characterized by spatial a shrinking of the wave and roll structure towards the pipe centre with increasing  $Re$, we continued the solution to
$Re$, we continued the solution to 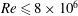 $Re\leqslant 8\times 10^{6}$ and we find only one unstable mode in the large Reynolds number regime, with growth rate scaling as
$Re\leqslant 8\times 10^{6}$ and we find only one unstable mode in the large Reynolds number regime, with growth rate scaling as 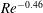 $Re^{-0.46}$ within the class of symmetry-preserving disturbances.
$Re^{-0.46}$ within the class of symmetry-preserving disturbances.
JFM classification
Information
- Type
- JFM Papers
- Information
- Copyright
- © 2019 Cambridge University Press
References
- 5
- Cited by

