Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Lee, Cunbiao
and
Jiang, Xianyang
2019.
Flow structures in transitional and turbulent boundary layers.
Physics of Fluids,
Vol. 31,
Issue. 11,
Schmidt, Oliver T.
2020.
Bispectral mode decomposition of nonlinear flows.
Nonlinear Dynamics,
Vol. 102,
Issue. 4,
p.
2479.
Gloerfelt, X.
Robinet, J.-C.
Sciacovelli, L.
Cinnella, P.
and
Grasso, F.
2020.
Dense-gas effects on compressible boundary-layer stability.
Journal of Fluid Mechanics,
Vol. 893,
Issue. ,
Ide, Yuki
Ito, Katsuhiro
and
Tanno, Hideyuki
2020.
Nonlinear resonance of low- and high-frequency waves in a cooled hypersonic boundary-layer.
Fluid Dynamics Research,
Vol. 52,
Issue. 5,
p.
055502.
Zhu, Wenkai
Chen, Xi
Zhu, Yiding
and
Lee, Cunbiao
2020.
Nonlinear interactions in the hypersonic boundary layer on the permeable wall.
Physics of Fluids,
Vol. 32,
Issue. 10,
Flood, John T.
Taubert, Lutz
and
Craig, Stuart A.
2020.
First and Mack-mode instabilities in a flat-plate boundary layer at Mach 4.
Unnikrishnan, S.
and
Gaitonde, Datta V.
2020.
Linear, nonlinear and transitional regimes of second-mode instability.
Journal of Fluid Mechanics,
Vol. 905,
Issue. ,
Xiong, Youde
Yu, Tao
Lin, Liquan
Zhao, Jiaquan
and
Wu, Jie
2020.
Nonlinear Instability Characterization of Hypersonic Laminar Boundary Layer.
AIAA Journal,
Vol. 58,
Issue. 12,
p.
5254.
Butler, Cameron S.
and
Laurence, Stuart J.
2021.
Interaction of second-mode wave packets with an axisymmetric expansion corner.
Experiments in Fluids,
Vol. 62,
Issue. 7,
Siddiqui, Farhan
Gragston, Mark
Saric, William S.
and
Bowersox, Rodney D. W.
2021.
Mack-mode instabilities on a cooled flared cone with discrete roughness elements at Mach 6.
Experiments in Fluids,
Vol. 62,
Issue. 10,
Zhang, Mingjie
Si, Wufei
and
Lee, Cunbiao
2021.
Heat Transfer and Recovery Factor of Aerodynamic Heating on a Flared Cone.
AIAA Journal,
Vol. 59,
Issue. 11,
p.
4284.
Siddiqui, Farhan
Saric, William S.
and
Bowersox, Rodney D.
2021.
Interaction of Second-Mode Disturbances and 3-D Roughness on a Cooled Flared Cone at Mach 6.
YU, Tao
XIONG, Youde
ZHAO, Jiaquan
and
WU, Jie
2021.
Application of focused laser differential interferometer to hypersonic boundary-layer instability study.
Chinese Journal of Aeronautics,
Vol. 34,
Issue. 5,
p.
17.
Groot, Koen J.
Patel, Jay
Saiyasak, Caleb
Coder, James G.
Stefanski, Douglas L.
and
Reed, Helen L.
2021.
Assessment of the Amplification Factor Transport Transition Model for High-Mach Number Flows.
Zhang, Chuanhong
and
Shi, Zhiwei
2021.
Nonlinear interactions in a hypersonic boundary layer.
AIP Advances,
Vol. 11,
Issue. 3,
Gragston, Mark
Siddiqui, Farhan
and
Schmisseur, John D.
2021.
Detection of second-mode instabilities on a flared cone in Mach 6 quiet flow with linear array focused laser differential interferometry.
Experiments in Fluids,
Vol. 62,
Issue. 4,
Zhu, Yiding
Gu, Dingwei
Zhu, Wenkai
Chen, Shiyi
Lee, Cunbiao
and
Oran, Elaine S.
2021.
Dilatational-wave-induced aerodynamic cooling in transitional hypersonic boundary layers.
Journal of Fluid Mechanics,
Vol. 911,
Issue. ,
Gragston, Mark
Siddiqui, Farhan
and
Schmisseur, John D.
2022.
Measurements of Second-Mode Instabilities with Linear Array-Focused Laser Differential Interferometry.
Zhao, Jiaquan
Sima, Xuehao
Xiong, Youde
Tu, Guohua
Li, Renfu
and
Wu, Jie
2022.
Impact of Wavy Wall Surface on Hypersonic Boundary-Layer Instability of Sharp Cone Model.
AIAA Journal,
Vol. 60,
Issue. 11,
p.
6203.
Pandey, Anshuman
Casper, Katya M.
Guildenbecher, Daniel R.
Beresh, Steven J.
Bhakta, Rajkumar
DeZetter, Marie E.
and
Spillers, Russell
2022.
Instability Measurements in Hypersonic Flow on a Three-Dimensional Cone-Slice-Ramp Geometry.
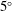 $5^{\circ }$ flared cone at
$5^{\circ }$ flared cone at 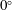 $0^{\circ }$ angle of attack in the low-disturbance Mach 6 Quiet Tunnel at Texas A&M University across a free-stream unit Reynolds number range of
$0^{\circ }$ angle of attack in the low-disturbance Mach 6 Quiet Tunnel at Texas A&M University across a free-stream unit Reynolds number range of  $7.8\times 10^{6}~\text{m}^{-1}\leqslant \mathit{Re}^{\prime }\leqslant 11.0\times 10^{6}~\text{m}^{-1}$. The high-frequency response of the measurement system allows harmonics and other nonlinear behaviour to be measured. Mack-mode waves and several harmonics are clearly observed at a frequency of
$7.8\times 10^{6}~\text{m}^{-1}\leqslant \mathit{Re}^{\prime }\leqslant 11.0\times 10^{6}~\text{m}^{-1}$. The high-frequency response of the measurement system allows harmonics and other nonlinear behaviour to be measured. Mack-mode waves and several harmonics are clearly observed at a frequency of 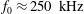 $f_{0}\approx 250~\text{kHz}$. Bispectral analysis is used to show that these waves undergo several quadratic phase-coupled sum and difference interactions with themselves to produce harmonics, as well interact with a relatively low-frequency wave that results in amplitude modulation. Bispectral analysis is used to highlight these interactions.
$f_{0}\approx 250~\text{kHz}$. Bispectral analysis is used to show that these waves undergo several quadratic phase-coupled sum and difference interactions with themselves to produce harmonics, as well interact with a relatively low-frequency wave that results in amplitude modulation. Bispectral analysis is used to highlight these interactions.