Article contents
Motion of a sphere in a viscous density stratified fluid
Published online by Cambridge University Press: 29 September 2022
Abstract
We examine the translation of a sphere in a stratified ambient in the limit of small Reynolds numbers ( $Re \ll 1$) and viscous Richardson numbers (
$Re \ll 1$) and viscous Richardson numbers ( $Ri_v \ll 1$); here,
$Ri_v \ll 1$); here,  $Re = {\rho Ua}/{\mu }$ and
$Re = {\rho Ua}/{\mu }$ and  $Ri_v = {\gamma a^3 g}/{\mu U}$, with
$Ri_v = {\gamma a^3 g}/{\mu U}$, with  $a$ being the sphere radius,
$a$ being the sphere radius,  $U$ the translation speed,
$U$ the translation speed,  $\rho$ and
$\rho$ and  $\mu$ the density and viscosity of the stratified ambient,
$\mu$ the density and viscosity of the stratified ambient,  $g$ the acceleration due to gravity, and
$g$ the acceleration due to gravity, and  $\gamma$ the density gradient characterizing the ambient stratification. In contrast to most earlier efforts, our study considers the convection-dominant limit corresponding to
$\gamma$ the density gradient characterizing the ambient stratification. In contrast to most earlier efforts, our study considers the convection-dominant limit corresponding to  $Pe = {Ua}/{D} \gg 1$,
$Pe = {Ua}/{D} \gg 1$,  $D$ being the diffusivity of the stratifying agent. We characterize in detail the velocity and density fields around the particle in what we term the Stokes stratification regime, defined by
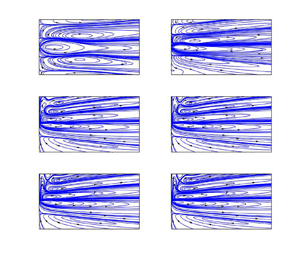
$D$ being the diffusivity of the stratifying agent. We characterize in detail the velocity and density fields around the particle in what we term the Stokes stratification regime, defined by  $Re \ll Ri_v^{{1}/{3}} \ll 1$, and corresponding to the dominance of buoyancy over inertial forces. Buoyancy forces associated with the perturbed stratification fundamentally alter the viscously dominated fluid motion at large distances of order the stratification screening length that scales as
$Re \ll Ri_v^{{1}/{3}} \ll 1$, and corresponding to the dominance of buoyancy over inertial forces. Buoyancy forces associated with the perturbed stratification fundamentally alter the viscously dominated fluid motion at large distances of order the stratification screening length that scales as  $a\,Ri_v^{-{1}/{3}}$. The motion at these distances transforms from the familiar fore–aft symmetric Stokesian form to a fore–aft asymmetric pattern of recirculating cells with primarily horizontal motion within, except in the vicinity of the rear stagnation streamline. At larger distances, the motion is vanishingly small except within (a) an axisymmetric horizontal wake whose vertical extent grows as
$a\,Ri_v^{-{1}/{3}}$. The motion at these distances transforms from the familiar fore–aft symmetric Stokesian form to a fore–aft asymmetric pattern of recirculating cells with primarily horizontal motion within, except in the vicinity of the rear stagnation streamline. At larger distances, the motion is vanishingly small except within (a) an axisymmetric horizontal wake whose vertical extent grows as  $O(r_t^{{2}/{5}})$,
$O(r_t^{{2}/{5}})$,  $r_t$ being the distance in the plane perpendicular to translation, and (b) a buoyant reverse jet behind the particle that narrows as the inverse square root of distance downstream. As a result, for
$r_t$ being the distance in the plane perpendicular to translation, and (b) a buoyant reverse jet behind the particle that narrows as the inverse square root of distance downstream. As a result, for  $Pe = \infty$, the motion close to the rear stagnation streamline starts off pointing in the direction of translation, in the inner region, and decaying as the inverse of the downstream distance; the motion reverses beyond distance
$Pe = \infty$, the motion close to the rear stagnation streamline starts off pointing in the direction of translation, in the inner region, and decaying as the inverse of the downstream distance; the motion reverses beyond distance  $1.15a\,Ri_v^{-{1}/{3}}$, with the eventual reverse flow in the far-field buoyant jet again decaying as the inverse of the distance downstream. For large but finite
$1.15a\,Ri_v^{-{1}/{3}}$, with the eventual reverse flow in the far-field buoyant jet again decaying as the inverse of the distance downstream. For large but finite  $Pe$, the narrowing jet is smeared out beyond a distance of
$Pe$, the narrowing jet is smeared out beyond a distance of  $O(a\,Ri_v^{-{1}/{2}}\, Pe^{{1}/{2}})$, leading to an exponential decay of the aforementioned reverse flow.
$O(a\,Ri_v^{-{1}/{2}}\, Pe^{{1}/{2}})$, leading to an exponential decay of the aforementioned reverse flow.
Information
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2022. Published by Cambridge University Press
References
REFERENCES
- 7
- Cited by


