Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Zabarankin, Michael
2016.
Liquid toroidal drop in compressional flow with arbitrary drop-to-ambient fluid viscosity ratio.
Proceedings of the Royal Society A: Mathematical, Physical and Engineering Sciences,
Vol. 472,
Issue. 2187,
p.
20150737.
Zabarankin, Michael
2017.
Liquid toroidal drop under uniform electric field.
Proceedings of the Royal Society A: Mathematical, Physical and Engineering Sciences,
Vol. 473,
Issue. 2202,
p.
20160633.
Fragkopoulos, A. A.
Aizenman, A.
and
Fernández-Nieves, A.
2017.
Charge-Induced Saffman-Taylor Instabilities in Toroidal Droplets.
Physical Review Letters,
Vol. 118,
Issue. 26,
Fragkopoulos, Alexandros A.
and
Fernández-Nieves, Alberto
2017.
Toroidal-droplet instabilities in the presence of charge.
Physical Review E,
Vol. 95,
Issue. 3,
Zabarankin, Michael
2017.
Toroidal drop under electric field: arbitrary drop-to-ambient fluid viscosity ratio.
Proceedings of the Royal Society A: Mathematical, Physical and Engineering Sciences,
Vol. 473,
Issue. 2205,
p.
20170379.
Zabarankin, Michael
and
Grechuk, Bogdan
2017.
Forward and Inverse Problems in Two-Phase Fluid Dynamics.
SIAM Journal on Control and Optimization,
Vol. 55,
Issue. 6,
p.
3969.
Fragkopoulos, Alexandros A.
Pairam, Ekapop
Berger, Eric
Segre, Phil N.
and
Fernández-Nieves, Alberto
2017.
Shrinking instability of toroidal droplets.
Proceedings of the National Academy of Sciences,
Vol. 114,
Issue. 11,
p.
2871.
Ee, B. K.
Lavrenteva, O. M.
Smagin, I.
and
Nir, A.
2018.
Evolution and stationarity of liquid toroidal drop in compressional Stokes flow.
Journal of Fluid Mechanics,
Vol. 835,
Issue. ,
p.
1.
Fragkopoulos, Alexandros A.
Pairam, Ekapop
Berger, Eric
and
Fernandez-Nieves, Alberto
2018.
Toroidal Droplets: Growth Rates, Dispersion Relations, and Behavior in the Thick-Torus Limit.
Langmuir,
Vol. 34,
Issue. 3,
p.
1218.
Leon Plata, Paola
Liu, Ying
and
Nitsche, Ludwig C.
2018.
Interaction of multiple drops and formation of toroidal-spiral particles.
Physical Review Fluids,
Vol. 3,
Issue. 9,
Fragkopoulos, Alexandros A.
Pairam, Ekapop
Marinkovic, Luka
and
Fernández-Nieves, Alberto
2018.
Breakup dynamics of toroidal droplets in shear-thinning fluids.
Physical Review E,
Vol. 97,
Issue. 2,
Zabarankin, Michael
2019.
Small Deformation Analysis for Stationary Toroidal Drops in a Compressional Flow.
SIAM Journal on Applied Mathematics,
Vol. 79,
Issue. 5,
p.
2150.
Zabarankin, Michael
2019.
A Leaky Dielectric Drop with Conical Ends in An Electric Field.
SIAM Journal on Applied Mathematics,
Vol. 79,
Issue. 5,
p.
1768.
Banerjee, Abhishek
Lavrenteva, Olga M.
Smagin, Irina
and
Nir, Avinoam
2021.
Viscoplastic toroidal drop in compressional Stokes flow.
Physics of Fluids,
Vol. 33,
Issue. 7,
Malik, Sumit
Lavrenteva, O.M.
and
Nir, A.
2021.
Dynamic and stationary shapes of rotating toroidal drops in viscous linear flows.
Journal of Fluid Mechanics,
Vol. 923,
Issue. ,
Lavrenteva, O.M.
Ee, B.K.
Smagin, I.
and
Nir, A.
2021.
Approximating stationary deformation of flat and toroidal drops in compressional viscous flow using generalized Cassini ovals.
Journal of Fluid Mechanics,
Vol. 921,
Issue. ,
Malik, Sumit
Lavrenteva, Olga M.
Idan, Moshe
and
Nir, Avinoam
2022.
Controlled stabilization of rotating toroidal drops in viscous linear flow.
Journal of Fluid Mechanics,
Vol. 952,
Issue. ,
Qin, Shiyi
Li, Zhaolin
Wang, Xun
Li, Kai
and
Chen, Xue
2022.
Numerical simulations of a toroidal droplet breakup in viscous oils.
AIP Advances,
Vol. 12,
Issue. 11,
Malik, Sumit
Lavrenteva, Olga M.
Idan, Moshe
and
Nir, Avinoam
2024.
Stationary dimpled drops under linear flow.
Journal of Fluid Mechanics,
Vol. 983,
Issue. ,
Li, Xiaonan
Li, Tianpeng
Xu, Tong
Zhao, Ran
Gao, Xinbao
and
Xuan, Zhaolong
2024.
A combined CFD-based simulation and experimental study of particle dispersion in a high-concentration airtight space under unorganized airflow.
Advances in Mechanical Engineering,
Vol. 16,
Issue. 2,
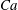 $Ca$ ), three different scenarios of drop evolution are possible: indefinite expansion of the liquid torus, contraction to the centre and a stationary toroidal shape. When the intensity of the flow is low, the stationary shapes are shown to be close to circular tori. Once the outer flow strengthens, the cross-section of the stationary torus assumes first an elliptic and then an egg-like shape. For the capillary number greater than a critical value,
$Ca$ ), three different scenarios of drop evolution are possible: indefinite expansion of the liquid torus, contraction to the centre and a stationary toroidal shape. When the intensity of the flow is low, the stationary shapes are shown to be close to circular tori. Once the outer flow strengthens, the cross-section of the stationary torus assumes first an elliptic and then an egg-like shape. For the capillary number greater than a critical value, 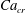 $Ca_{cr}$ , toroidal stationary shapes were not found. Remarkably,
$Ca_{cr}$ , toroidal stationary shapes were not found. Remarkably, 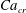 $Ca_{cr}$ is close to the critical capillary number found previously for a simply connected drop flattened in compressional flow. Thus, a new example of non-uniqueness of stationary drop shape in viscous flow is obtained. Approximate stationary solutions in the form of tori with circular and elliptic cross-sections are obtained by minimizing the normal velocity over the drop interface. They are shown to be in good agreement with the stationary shapes from quasi-dynamic simulations for the corresponding intervals of the capillary number.
$Ca_{cr}$ is close to the critical capillary number found previously for a simply connected drop flattened in compressional flow. Thus, a new example of non-uniqueness of stationary drop shape in viscous flow is obtained. Approximate stationary solutions in the form of tori with circular and elliptic cross-sections are obtained by minimizing the normal velocity over the drop interface. They are shown to be in good agreement with the stationary shapes from quasi-dynamic simulations for the corresponding intervals of the capillary number.

