Hostname: page-component-745bb68f8f-f46jp Total loading time: 0 Render date: 2025-01-10T21:56:11.234Z Has data issue: false hasContentIssue false
  • English
  • Français

Large-eddy simulation of high-Reynolds-number turbulent channel flow controlled using streamwise travelling wave-like wall deformation for drag reduction

Published online by Cambridge University Press:  10 January 2025

Yusuke Nabae Open the ORCID record for Yusuke Nabae [Opens in a new window] ,
Kazuhiro Inagaki Open the ORCID record for Kazuhiro Inagaki [Opens in a new window] ,
Hiromichi Kobayashi Open the ORCID record for Hiromichi Kobayashi [Opens in a new window] ,
Hiroshi Gotoda Open the ORCID record for Hiroshi Gotoda [Opens in a new window]  and
Koji Fukagata Open the ORCID record for Koji Fukagata [Opens in a new window]
Yusuke Nabae*
Affiliation:
Department of Mechanical Engineering, Tokyo University of Science, 6-3-1 Niijuku, Katsushika-ku, Tokyo 125-8585, Japan
Kazuhiro Inagaki
Affiliation:
Department of Mechanical Engineering, Doshisha University, 1-3 Tatara Miyakodani, Kyotanabe 610-0394, Japan
Hiromichi Kobayashi
Affiliation:
Department of Physics & Research and Education Center for Natural Sciences, Keio University, 4-1-1 Hiyoshi, Kohoku-ku, Yokohama 223-8521, Japan
Hiroshi Gotoda
Affiliation:
Department of Mechanical Engineering, Tokyo University of Science, 6-3-1 Niijuku, Katsushika-ku, Tokyo 125-8585, Japan
Koji Fukagata
Affiliation:
Department of Mechanical Engineering, Keio University, 3-14-1 Hiyoshi, Kohoku-ku, Yokohama 223-8522, Japan
*
†Email address for correspondence: yusuke.nabae@rs.tus.ac.jp
Get access Rights & Permissions [Opens in a new window]

Abstract

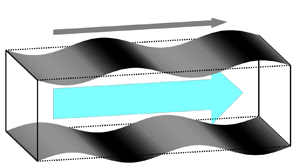
We investigate the drag reduction effect of the streamwise travelling wave-like wall deformation in a high-Reynolds-number turbulent channel flow by large-eddy simulation (LES). First, we assess the validity of subgrid-scale models in uncontrolled and controlled flows. For friction Reynolds numbers $Re_\tau = 360$ and $720$, the Smagorinsky and wall-adapting local eddy-viscosity (WALE) models with a damping function can reproduce well the mean velocity profile obtained by direct numerical simulation (DNS) in both the uncontrolled and controlled flows, leading to a small difference in drag reduction rate between LES and DNS. The LES with finer grid resolution can reproduce well the key structures observed in the DNS of the controlled flow. These results show that the high-fidelity LES is valid for appropriately predicting the drag reduction effect. In addition, a small computational domain is sufficient for reproducing the turbulence statistics, key structures and drag reduction rate obtained by DNS. Subsequently, to investigate the trend of drag reduction rate at higher Reynolds numbers, we utilize the WALE model with the damping function to investigate the control effect at higher Reynolds numbers up to $Re_\tau = 3240$. According to the analyses of turbulence statistics and instantaneous flow fields, the drag reduction at higher Reynolds numbers occurs basically through the same mechanism as that at lower Reynolds numbers. In addition, the drag reduction rate obtained by the present LES approaches that predicted using the semi-empirical formula (Nabae et al., Intl J. Heat Fluid Flow, vol. 82, 2020, 108550) as the friction Reynolds number increases, which supports the high predictability of the semi-empirical formula at significantly high Reynolds numbers.

JFM classification

Flow Control: Drag reduction Turbulent Flows: Turbulence control Waves/Free-surface Flows: Channel flow
Type
JFM Papers
Information
Journal of Fluid Mechanics , Volume 1003 , 25 January 2025 , A2
Check for updates
Copyright
© The Author(s), 2025. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Abe, H., Kawamura, H. & Matsuo, Y. 2004 Surface heat-flux fluctuations in a turbulent channel flow up to ${Re}_\tau =1020$ with ${Pr}= 0.025$ and $0.71$. Intl J. Heat Fluid Flow 25 (3), 404–419.CrossRefGoogle Scholar
Albers, M., Shao, X. & Schröder, W. 2024 Energy efficient actuated drag reduced compressible turbulent flat plate flow. Intl J. Heat Fluid Flow 106, 109314.CrossRefGoogle Scholar
Bernardini, M., Pirozzoli, S. & Orlandi, P. 2014 Velocity statistics in turbulent channel flow up to ${Re}_\tau = 4000$. J. Fluid Mech. 742, 171–191.CrossRefGoogle Scholar
Chang, Y., Collis, S.S. & Ramakrishnan, S. 2002 Viscous effects in control of near-wall turbulence. Phys. Fluids 14 (11), 4069–4080.CrossRefGoogle Scholar
Choi, H., Moin, P. & Kim, J. 1994 Active turbulence control for drag reduction in wall-bounded flows. J. Fluid Mech. 262, 75–110.CrossRefGoogle Scholar
Cimarelli, A. & De Angelis, E. 2012 Anisotropic dynamics and sub-grid energy transfer in wall-turbulence. Phys. Fluids 24 (1), 015102.CrossRefGoogle Scholar
Dukowicz, J.K. & Dvinsky, A.S. 1992 Approximate factorization as a high order splitting for the implicit incompressible flow equations. J. Comput. Phys. 102 (2), 336–347.CrossRefGoogle Scholar
Fukagata, K., Iwamoto, K. & Hasegawa, Y. 2024 Turbulent drag reduction by streamwise traveling waves of wall-normal forcing. Annu. Rev. Fluid Mech. 56, 69–90.CrossRefGoogle Scholar
Fukagata, K., Kasagi, N. & Koumoutsakos, P. 2006 A theoretical prediction of friction drag reduction in turbulent flow by superhydrophobic surfaces. Phys. Fluids 18 (5), 051703.CrossRefGoogle Scholar
Gad-el-Hak, M. 1994 Interactive control of turbulent boundary layers: a futuristic overview. AIAA J. 32 (9), 1753–1765.CrossRefGoogle Scholar
Gatti, D. & Quadrio, M. 2016 Reynolds-number dependence of turbulent skin-friction drag reduction induced by spanwise forcing. J. Fluid Mech. 802, 553–582.CrossRefGoogle Scholar
Germano, M., Piomelli, U., Moin, P. & Cabot, W.H. 1991 A dynamic subgrid-scale eddy viscosity model. Phys. Fluids 3 (7), 1760–1765.CrossRefGoogle Scholar
Hoyas, S. & Jiménez, J. 2006 Scaling of the velocity fluctuations in turbulent channels up to ${Re}_\tau = 2003$. Phys. Fluids 18 (1), 011702.CrossRefGoogle Scholar
Hoyas, S., Oberlack, M., Alcántara-Ávila, F., Kraheberger, S.V. & Laux, J. 2022 Wall turbulence at high friction Reynolds numbers. Phys. Rev. Fluids 7 (1), 014602.CrossRefGoogle Scholar
Hunt, J.C.R., Wray, A.A. & Moin, P. 1988 Eddies, streams, and convergence zones in turbulent flows. Center for Turbulence Research Report, CTR-S88.Google Scholar
Hurst, E., Yang, Q. & Chung, Y.M. 2014 The effect of Reynolds number on turbulent drag reduction by streamwise travelling waves. J. Fluid Mech. 759, 28–55.CrossRefGoogle Scholar
Hussain, A.K.M.F. & Reynolds, W.C. 1970 The mechanics of an organized wave in turbulent shear flow. J. Fluid Mech. 41 (2), 241–258.CrossRefGoogle Scholar
Hwang, Y. & Cossu, C. 2010 Self-sustained process at large scales in turbulent channel flow. Phys. Rev. Lett. 105 (4), 044505.CrossRefGoogle Scholar
Iwamoto, K., Fukagata, K., Kasagi, N. & Suzuki, Y. 2005 Friction drag reduction achievable by near-wall turbulence manipulation at high Reynolds numbers. Phys. Fluids 17 (1), 011702.CrossRefGoogle Scholar
Iwamoto, K., Suzuki, Y. & Kasagi, N. 2002 Reynolds number effect on wall turbulence: toward effective feedback control. Intl J. Heat Fluid Flow 23 (5), 678–689.CrossRefGoogle Scholar
Kang, S. & Choi, H. 2000 Active wall motions for skin-friction drag reduction. Phys. Fluids 12 (12), 3301–3304.CrossRefGoogle Scholar
Kasagi, N., Suzuki, Y. & Fukagata, K. 2009 Microelectromechanical systems–based feedback control of turbulence for skin friction reduction. Annu. Rev. Fluid Mech. 41, 231–251.CrossRefGoogle Scholar
Kawagoe, A., Nakashima, S., Luhar, M. & Fukagata, K. 2019 Proposal of control laws for turbulent skin friction reduction based on resolvent analysis. J. Fluid Mech. 866, 810–840.CrossRefGoogle Scholar
Kim, J. 2003 Control of turbulent boundary layers. Phys. Fluids 15 (5), 1093–1105.CrossRefGoogle Scholar
Kim, J. & Bewley, T.R. 2007 A linear systems approach to flow control. Annu. Rev. Fluid Mech. 39, 383–417.CrossRefGoogle Scholar
Koganezawa, S., Mitsuishi, A., Shimura, T., Iwamoto, K., Mamori, H. & Murata, A. 2019 Pathline analysis of traveling wavy blowing and suction control in turbulent pipe flow for drag reduction. Intl J. Heat Fluid Flow 77, 388–401.CrossRefGoogle Scholar
Krug, D., Philip, J. & Marusic, I. 2017 Revisiting the law of the wake in wall turbulence. J. Fluid Mech. 811, 421–435.CrossRefGoogle Scholar
Lee, C., Kim, J. & Choi, H. 1998 Suboptimal control of turbulent channel flow for drag reduction. J. Fluid Mech. 358, 245–258.CrossRefGoogle Scholar
Lee, C., Min, T. & Kim, J. 2008 Stability of a channel flow subject to wall blowing and suction in the form of a traveling wave. Phys. Fluids 20 (10), 101513.CrossRefGoogle Scholar
Lee, M. & Moser, R.D. 2015 Direct numerical simulation of turbulent channel flow up to ${Re}_\tau \approx 5200$. J. Fluid Mech. 774, 395–415.CrossRefGoogle Scholar
Lieu, B.K., Moarref, R. & Jovanović, M.R. 2010 Controlling the onset of turbulence by streamwise travelling waves. Part 2. Direct numerical simulation. J. Fluid Mech. 663, 100–119.CrossRefGoogle Scholar
Lilly, D.K. 1992 A proposed modification of the Germano subgrid-scale closure method. Phys. Fluids 4 (3), 633–635.CrossRefGoogle Scholar
Mamori, H., Fukudome, K., Ogino, K., Fukushima, N. & Yamamoto, M. 2021 Heat transfer enhancement and torque reduction by traveling wave-like blowing and suction in turbulent Taylor–Couette flow. J. Therm. Sci. Technol. 16 (1), JTST0003.CrossRefGoogle Scholar
Mamori, H., Iwamoto, K. & Murata, A. 2014 Effect of the parameters of traveling waves created by blowing and suction on the relaminarization phenomena in fully developed turbulent channel flow. Phys. Fluids 26 (1), 015101.CrossRefGoogle Scholar
Marusic, I., Chandran, D., Rouhi, A., Fu, M.K., Wine, D., Holloway, B., Chung, D. & Smits, A.J. 2021 An energy-efficient pathway to turbulent drag reduction. Nat. Commun. 12 (1), 5805.CrossRefGoogle ScholarPubMed
Meneveau, C. & Katz, J. 2000 Scale-invariance and turbulence models for large-eddy simulation. Annu. Rev. Fluid Mech. 32 (1), 1–32.CrossRefGoogle Scholar
Min, T., Kang, S.M., Speyer, J.L. & Kim, J. 2006 Sustained sub-laminar drag in a fully developed channel flow. J. Fluid Mech. 558, 309–318.CrossRefGoogle Scholar
Moarref, R. & Jovanović, M.R. 2010 Controlling the onset of turbulence by streamwise travelling waves. Part 1. Receptivity analysis. J. Fluid Mech. 663, 70–99.CrossRefGoogle Scholar
Morinishi, Y., Lund, T.S., Vasilyev, O.V. & Moin, P. 1998 Fully conservative higher order finite difference schemes for incompressible flow. J. Comput. Phys. 143 (1), 90–124.CrossRefGoogle Scholar
Moser, R.D., Haering, S.W. & Yalla, G.R. 2021 Statistical properties of subgrid-scale turbulence models. Annu. Rev. Fluid Mech 53, 255–286.CrossRefGoogle Scholar
Murai, Y. 2014 Frictional drag reduction by bubble injection. Exp. Fluids 55 (7), 1–28.CrossRefGoogle Scholar
Nabae, Y. & Fukagata, K. 2021 Bayesian optimization of traveling wave-like wall deformation for friction drag reduction in turbulent channel flow. J. Fluid Sci. Technol. 16 (4), JFST0024.CrossRefGoogle Scholar
Nabae, Y. & Fukagata, K. 2022 Drag reduction effect of streamwise traveling wave-like wall deformation with spanwise displacement variation in turbulent channel flow. Flow Turbul. Combust. 109 (4), 1175–1194.CrossRefGoogle Scholar
Nabae, Y. & Fukagata, K. 2024 Theoretical and numerical analyses of turbulent plane Couette flow controlled using uniform blowing and suction. Intl J. Heat Fluid Flow 106, 109286.CrossRefGoogle Scholar
Nabae, Y., Kawai, K. & Fukagata, K. 2020 Prediction of drag reduction effect by streamwise traveling wave-like wall deformation in turbulent channel flow at practically high reynolds numbers. Intl J. Heat Fluid Flow 82, 108550.CrossRefGoogle Scholar
Nakanishi, R., Mamori, H. & Fukagata, K. 2012 Relaminarization of turbulent channel flow using traveling wave-like wall deformation. Intl J. Heat Fluid Flow 35, 152–159.CrossRefGoogle Scholar
Nakashima, S., Fukagata, K. & Luhar, M. 2017 Assessment of suboptimal control for turbulent skin friction reduction via resolvent analysis. J. Fluid Mech. 828, 496–526.CrossRefGoogle Scholar
Nicoud, F. & Ducros, F. 1999 Subgrid-scale stress modelling based on the square of the velocity gradient tensor. Flow Turbul. Combust. 62 (3), 183–200.CrossRefGoogle Scholar
Ogino, K., Mamori, H., Fukushima, N., Fukudome, K. & Yamamoto, M. 2019 Direct numerical simulation of Taylor–Couette turbulent flow controlled by a traveling wave-like blowing and suction. Intl J. Heat Fluid Flow 80, 108463.CrossRefGoogle Scholar
Pope, S.B. 2000 Turbulent Flows. Cambridge University Press.Google Scholar
Reynolds, W.C. & Tiederman, W.G. 1967 Stability of turbulent channel flow, with application to Malkus's theory. J. Fluid Mech. 27 (2), 253–272.CrossRefGoogle Scholar
Ricco, P., Skote, M. & Leschziner, M.A. 2021 A review of turbulent skin-friction drag reduction by near-wall transverse forcing. Prog. Aerosp. Sci. 123, 100713.CrossRefGoogle Scholar
Rouhi, A., Fu, M.K., Chandran, D., Zampiron, A., Smits, A.J. & Marusic, I. 2023 Turbulent drag reduction by spanwise wall forcing. Part 1. Large-eddy simulations. J. Fluid Mech. 968, A6.CrossRefGoogle Scholar
Smagorinsky, J. 1963 General circulation experiments with the primitive equations. Mon. Weath. Rev. 91 (3), 99–164.2.3.CO;2>CrossRefGoogle Scholar
Spalart, P.R., Moser, R.D. & Rogers, M.M. 1991 Spectral methods for the Navier–Stokes equations with one infinite and two periodic directions. J. Comput. Phys. 96 (2), 297–324.CrossRefGoogle Scholar
Suzuki, I., Shimura, T., Mitsuishi, A., Iwamoto, K. & Murata, A. 2019 Experimental study on drag reduction effect with traveling wave control using PIV measurement. In Proceeding of ASME-JSME-KSME Joint Fluids Engineering Conference 2019, San Francisco, USA, AJKFLUID2019-4855.Google Scholar
Walsh, M.J. 1983 Riblets as a viscous drag reduction technique. AIAA J. 21 (4), 485–486.CrossRefGoogle Scholar
White, C.M. & Mungal, M.G. 2008 Mechanics and prediction of turbulent drag reduction with polymer additives. Annu. Rev. Fluid Mech. 40, 235–256.CrossRefGoogle Scholar
Yamamoto, Y. & Tsuji, Y. 2018 Numerical evidence of logarithmic regions in channel flow at ${Re}_\tau = 8000$. Phys. Rev. Fluids 3 (1), 012602.CrossRefGoogle Scholar