Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Klettner, Christian A.
and
Eames, Ian
2012.
Momentum and energy of a solitary wave interacting with a submerged semi-circular cylinder.
Journal of Fluid Mechanics,
Vol. 708,
Issue. ,
p.
576.
Klettner, C.A.
and
Eames, I.
2013.
Viscous free surface simulations with the characteristic based split scheme.
Computers & Fluids,
Vol. 71,
Issue. ,
p.
487.
Klettner, C. A.
and
Eames, I.
2015.
Low-Reynolds-number flow past a cylinder with uniform blowing or sucking.
Journal of Fluid Mechanics,
Vol. 780,
Issue. ,
Klettner, C. A.
Eames, I.
Semsarzadeh, S.
and
Nicolle, A.
2016.
The effect of a uniform through-surface flow on a cylinder and sphere.
Journal of Fluid Mechanics,
Vol. 793,
Issue. ,
p.
798.
Önder, Asim
and
Liu, Philip L.-F.
2020.
Stability of the solitary wave boundary layer subject to finite-amplitude disturbances.
Journal of Fluid Mechanics,
Vol. 896,
Issue. ,
Bogucki, Darek J.
Haus, Brian K.
Barzegar, Mohammad
Shao, Mingming
and
Domaradzki, Julian A.
2020.
On the Nature of the Turbulent Energy Dissipation Beneath Nonbreaking Waves.
Geophysical Research Letters,
Vol. 47,
Issue. 19,
Bogucki, Darek J.
Haus, Brian K.
and
Barzegar, Mohammad
2022.
Observations and Parametrization of the Turbulent Energy Dissipation Beneath Non-Breaking Waves.
Fluids,
Vol. 7,
Issue. 7,
p.
216.
Deb, Purbasha
and
Layek, Gorachand
2025.
Solitons at deformable free surface of nano‐fluid layer heated underneath.
ZAMM - Journal of Applied Mathematics and Mechanics / Zeitschrift für Angewandte Mathematik und Mechanik,
Vol. 105,
Issue. 5,

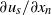 and
and 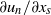 are comparable in magnitude, where
are comparable in magnitude, where  is the velocity,
is the velocity,  position and subscripts
position and subscripts  and
and  refer to components tangential and normal to the free surface. In this region
refer to components tangential and normal to the free surface. In this region 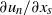 is approximately constant across the boundary layer while
is approximately constant across the boundary layer while 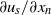 varies with
varies with 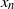 and outside the boundary layer tends to
and outside the boundary layer tends to 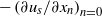 . The numerical results are compared to analytical models and good agreement is found.
. The numerical results are compared to analytical models and good agreement is found.