Published online by Cambridge University Press: 24 January 2019
Mixing of several species in high-pressure (high-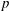 $p$) turbulent flows is investigated to understand the influence of the number of species on the flow characteristics. Direct numerical simulations are conducted in the temporal mixing layer configuration at approximately the same value of the momentum ratio for all realizations. The simulations are performed with mixtures of two, three, five and seven species to address various compositions at fixed number of species, at three values of initial vorticity-thickness-based Reynolds number,
$p$) turbulent flows is investigated to understand the influence of the number of species on the flow characteristics. Direct numerical simulations are conducted in the temporal mixing layer configuration at approximately the same value of the momentum ratio for all realizations. The simulations are performed with mixtures of two, three, five and seven species to address various compositions at fixed number of species, at three values of initial vorticity-thickness-based Reynolds number, 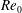 $Re_{0}$, and two values of the free-stream pressure,
$Re_{0}$, and two values of the free-stream pressure, 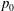 $p_{0}$, which is supercritical for each species except water. The major species are C7H16, O2 and N2, and the minor species are CO, CO2, H2 and H2O. The extensive database thus obtained allows the study of the influence not only of
$p_{0}$, which is supercritical for each species except water. The major species are C7H16, O2 and N2, and the minor species are CO, CO2, H2 and H2O. The extensive database thus obtained allows the study of the influence not only of 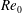 $Re_{0}$ and
$Re_{0}$ and 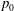 $p_{0}$, but also of the initial density ratio and of the initial density difference between streams,
$p_{0}$, but also of the initial density ratio and of the initial density difference between streams,  $\unicode[STIX]{x0394}\unicode[STIX]{x1D70C}$. The results show that the layer growth is practically insensitive to all of the above parameters; however, global vortical aspects increase with
$\unicode[STIX]{x0394}\unicode[STIX]{x1D70C}$. The results show that the layer growth is practically insensitive to all of the above parameters; however, global vortical aspects increase with  $Re_{0},p_{0}$ and the number of species; nevertheless, at the same
$Re_{0},p_{0}$ and the number of species; nevertheless, at the same 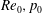 $Re_{0},p_{0}$ and density ratio, vorticity aspects are not influenced by the number of species. Species mixing produces strong density gradients which increase with
$Re_{0},p_{0}$ and density ratio, vorticity aspects are not influenced by the number of species. Species mixing produces strong density gradients which increase with 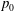 $p_{0}$ and otherwise scale with
$p_{0}$ and otherwise scale with 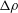 $\unicode[STIX]{x0394}\unicode[STIX]{x1D70C}$ but, when scaled by
$\unicode[STIX]{x0394}\unicode[STIX]{x1D70C}$ but, when scaled by 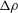 $\unicode[STIX]{x0394}\unicode[STIX]{x1D70C}$, are not affected by the number of species. Generalized Korteweg-type equations are developed for a multi-species mixture, and a priori estimates based on the largest density gradient show that the Korteweg stresses, which account for the influence of the density gradient, have negligible contribution in the momentum equation. The species-specific effective Schmidt number,
$\unicode[STIX]{x0394}\unicode[STIX]{x1D70C}$, are not affected by the number of species. Generalized Korteweg-type equations are developed for a multi-species mixture, and a priori estimates based on the largest density gradient show that the Korteweg stresses, which account for the influence of the density gradient, have negligible contribution in the momentum equation. The species-specific effective Schmidt number, 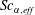 $Sc_{\unicode[STIX]{x1D6FC},\mathit{eff}}$, is computed and it is found that negative values occur for all minor species – particularly for H2 – thus indicating uphill diffusion, while the major species experience only regular diffusion. The probability density function (p.d.f.) of
$Sc_{\unicode[STIX]{x1D6FC},\mathit{eff}}$, is computed and it is found that negative values occur for all minor species – particularly for H2 – thus indicating uphill diffusion, while the major species experience only regular diffusion. The probability density function (p.d.f.) of 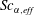 $Sc_{\unicode[STIX]{x1D6FC},\mathit{eff}}$ shows strong variation with
$Sc_{\unicode[STIX]{x1D6FC},\mathit{eff}}$ shows strong variation with  $p_{0}$ but weak dependence on the number of species; however, the p.d.f. substantially varies with the identity of the species. In contrast, the p.d.f. of the effective Prandtl number indicates dependence on both
$p_{0}$ but weak dependence on the number of species; however, the p.d.f. substantially varies with the identity of the species. In contrast, the p.d.f. of the effective Prandtl number indicates dependence on both 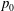 $p_{0}$ and the number of species. Similar to
$p_{0}$ and the number of species. Similar to 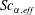 $Sc_{\unicode[STIX]{x1D6FC},\mathit{eff}}$, the species-specific effective Lewis-number p.d.f. depends on the species, and for all species the mean is smaller than unity, thus invalidating one of the most popular assumptions in combustion modelling. Simplifying the mixture composition by reducing the number of minor species does not affect the crucial species–temperature relationship of the major species that, for accuracy, must be retained in combustion simulations, but this relationship is affected for the minor species and in regions of uphill diffusion, indicating that the reduction is nonlinear in nature.
$Sc_{\unicode[STIX]{x1D6FC},\mathit{eff}}$, the species-specific effective Lewis-number p.d.f. depends on the species, and for all species the mean is smaller than unity, thus invalidating one of the most popular assumptions in combustion modelling. Simplifying the mixture composition by reducing the number of minor species does not affect the crucial species–temperature relationship of the major species that, for accuracy, must be retained in combustion simulations, but this relationship is affected for the minor species and in regions of uphill diffusion, indicating that the reduction is nonlinear in nature.