Article contents
Inertial wave excitation and focusing in a liquid bounded by a frustum and a cylinder
Published online by Cambridge University Press: 18 June 2014
Abstract
The mechanism of localized inertial wave excitation and its efficiency is investigated for an annular cavity rotating with  $\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}\Omega _0$ . Meridional symmetry is broken by replacing the inner cylinder with a truncated cone (frustum). Waves are excited by individual longitudinal libration of the walls. The geometry is non-separable and exhibits wave focusing and wave attractors. We investigated laboratory and numerical results for the Ekman number
$\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}\Omega _0$ . Meridional symmetry is broken by replacing the inner cylinder with a truncated cone (frustum). Waves are excited by individual longitudinal libration of the walls. The geometry is non-separable and exhibits wave focusing and wave attractors. We investigated laboratory and numerical results for the Ekman number 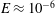 $E\approx 10^{-6}$ , inclination
$E\approx 10^{-6}$ , inclination  $\alpha =5.71^\circ $ and libration amplitudes
$\alpha =5.71^\circ $ and libration amplitudes 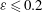 $\varepsilon \leq 0.2$ within the inertial wave band
$\varepsilon \leq 0.2$ within the inertial wave band  $0 < \omega < 2\Omega _0$ . Under the assumption that the inertial waves do not essentially affect the boundary-layer structure, we use classical boundary-layer analysis to study oscillating Ekman layers over a librating wall that is at an angle
$0 < \omega < 2\Omega _0$ . Under the assumption that the inertial waves do not essentially affect the boundary-layer structure, we use classical boundary-layer analysis to study oscillating Ekman layers over a librating wall that is at an angle 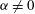 $\alpha \neq 0$ to the axis of rotation. The Ekman layer erupts at frequency
$\alpha \neq 0$ to the axis of rotation. The Ekman layer erupts at frequency 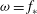 $\omega =f_{*}$ , where
$\omega =f_{*}$ , where 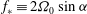 $f_{*}\equiv 2 \Omega _0 \sin \alpha $ is the effective Coriolis parameter in a plane tangential to the wall. For the selected inclination this eruption occurs for the forcing frequency
$f_{*}\equiv 2 \Omega _0 \sin \alpha $ is the effective Coriolis parameter in a plane tangential to the wall. For the selected inclination this eruption occurs for the forcing frequency 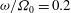 $\omega /\Omega _0=0.2$ . For the librating lids eruption occurs at
$\omega /\Omega _0=0.2$ . For the librating lids eruption occurs at 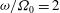 $\omega /\Omega _0=2$ . The study reveals that the frequency dependence of the total kinetic energy
$\omega /\Omega _0=2$ . The study reveals that the frequency dependence of the total kinetic energy  $K_{\omega }$ of the excited wave field is strongly connected to the square of the Ekman pumping velocity
$K_{\omega }$ of the excited wave field is strongly connected to the square of the Ekman pumping velocity 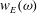 $w_{{E}}(\omega )$ that, in the linear limit, becomes singular when the boundary layer erupts. This explains the frequency dependence of non-resonantly excited waves. By the localization of the forcing, the two configurations investigated, (i) frustum libration and (ii) lids together with outer cylinder in libration, can be clearly distinguished by their response spectra. Good agreement was found for the spatial structure of low-order wave attractors and periodic orbits (both characterized by a small number of reflections) in the frequency windows predicted by geometric ray tracing. For ‘resonant’ frequencies a significantly increased total bulk energy was found, while the energy in the boundary layer remained nearly constant. Inertial wave energy enters the bulk flow via corner beams, which are parallel to the characteristics of the underlying Poincaré problem. Numerical simulations revealed a mismatch between the wall-parallel mass fluxes near the corners. This leads to boundary-layer eruption and the generation of inertial waves in the corners.
$w_{{E}}(\omega )$ that, in the linear limit, becomes singular when the boundary layer erupts. This explains the frequency dependence of non-resonantly excited waves. By the localization of the forcing, the two configurations investigated, (i) frustum libration and (ii) lids together with outer cylinder in libration, can be clearly distinguished by their response spectra. Good agreement was found for the spatial structure of low-order wave attractors and periodic orbits (both characterized by a small number of reflections) in the frequency windows predicted by geometric ray tracing. For ‘resonant’ frequencies a significantly increased total bulk energy was found, while the energy in the boundary layer remained nearly constant. Inertial wave energy enters the bulk flow via corner beams, which are parallel to the characteristics of the underlying Poincaré problem. Numerical simulations revealed a mismatch between the wall-parallel mass fluxes near the corners. This leads to boundary-layer eruption and the generation of inertial waves in the corners.
Information
- Type
- Papers
- Information
- Copyright
- © 2014 Cambridge University Press
References
- 34
- Cited by

