Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Ledesma-Aguilar, Rodrigo
Vella, Dominic
and
Yeomans, Julia M.
2014.
Lattice-Boltzmann simulations of droplet evaporation.
Soft Matter,
Vol. 10,
Issue. 41,
p.
8267.
Trinh, Philippe H.
Wilson, Stephen K.
and
Stone, Howard A.
2014.
A pinned or free-floating rigid plate on a thin viscous film.
Journal of Fluid Mechanics,
Vol. 760,
Issue. ,
p.
407.
Pruessner, Luisa
and
Smith, Frank
2015.
Enhanced effects from tiny flexible in-wall blips and shear flow.
Journal of Fluid Mechanics,
Vol. 772,
Issue. ,
p.
16.
Wei, Z.
Schneider, T. M.
Kim, J.
Kim, H.-Y.
Aizenberg, J.
and
Mahadevan, L.
2015.
Elastocapillary coalescence of plates and pillars.
Proceedings of the Royal Society A: Mathematical, Physical and Engineering Sciences,
Vol. 471,
Issue. 2175,
p.
20140593.
Hadjittofis, Andreas
Lister, John R.
Singh, Kiran
and
Vella, Dominic
2016.
Evaporation effects in elastocapillary aggregation.
Journal of Fluid Mechanics,
Vol. 792,
Issue. ,
p.
168.
Kim, Tae-Hong
Kim, Jungchul
and
Kim, Ho-Young
2016.
Evaporation-driven clustering of microscale pillars and lamellae.
Physics of Fluids,
Vol. 28,
Issue. 2,
Matia, Yoav
Elimelech, Tsah
and
Gat, Amir D.
2017.
Leveraging Internal Viscous Flow to Extend the Capabilities of Beam-Shaped Soft Robotic Actuators.
Soft Robotics,
Vol. 4,
Issue. 2,
p.
126.
Kodio, Ousmane
Griffiths, Ian M.
and
Vella, Dominic
2017.
Lubricated wrinkles: Imposed constraints affect the dynamics of wrinkle coarsening.
Physical Review Fluids,
Vol. 2,
Issue. 1,
Dersoir, B.
Schofield, A. B.
and
Tabuteau, H.
2017.
Clogging transition induced by self filtration in a slit pore.
Soft Matter,
Vol. 13,
Issue. 10,
p.
2054.
Fei, Yun (Raymond)
Maia, Henrique Teles
Batty, Christopher
Zheng, Changxi
and
Grinspun, Eitan
2017.
A multi-scale model for simulating liquid-hair interactions.
ACM Transactions on Graphics,
Vol. 36,
Issue. 4,
p.
1.
Sauret, Alban
Boulogne, François
Somszor, Katarzyna
Dressaire, Emilie
and
Stone, Howard A.
2017.
Drop morphologies on flexible fibers: influence of elastocapillary effects.
Soft Matter,
Vol. 13,
Issue. 1,
p.
134.
Bico, José
Reyssat, Étienne
and
Roman, Benoît
2018.
Elastocapillarity: When Surface Tension Deforms Elastic Solids.
Annual Review of Fluid Mechanics,
Vol. 50,
Issue. 1,
p.
629.
Shin, Dongwoo
and
Tawfick, Sameh
2018.
Polymorphic Elastocapillarity: Kinetically Reconfigurable Self-Assembly of Hair Bundles by Varying the Drain Rate.
Langmuir,
Vol. 34,
Issue. 21,
p.
6231.
Takahashi, Kosuke
Matsuo, Takahiro
Inaba, Kazuaki
and
Kishimoto, Kikuo
2019.
Bending Deformation of Multiple Aligned Plates Toward Coalescence Induced by Capillarity.
Journal of Microelectromechanical Systems,
Vol. 28,
Issue. 4,
p.
685.
Nasouri, Babak
Thorne, Benjamin
and
Elfring, Gwynn J.
2019.
Dynamics of poroelastocapillary rise.
Journal of Fluids and Structures,
Vol. 85,
Issue. ,
p.
220.
Vrancken, Nandi
Ghosh, Tanmay
Anand, Utkarsh
Aabdin, Zainul
Chee, See Wee
Baraissov, Zhaslan
Terryn, Herman
Gendt, Stefan De
Tao, Zheng
Xu, XiuMei
Holsteyns, Frank
and
Mirsaidov, Utkur
2020.
Nanoscale Elastocapillary Effect Induced by Thin-Liquid-Film Instability.
The Journal of Physical Chemistry Letters,
Vol. 11,
Issue. 7,
p.
2751.
Boyko, Evgeniy
Eshel, Ran
Gat, Amir D.
and
Bercovici, Moran
2020.
Nonuniform Electro-osmotic Flow Drives Fluid-Structure Instability.
Physical Review Letters,
Vol. 124,
Issue. 2,
Garcia-Gonzalez, Diana
Snoeijer, Jacco
Kappl, Michael
and
Butt, Hans-Jürgen
2020.
Onset of Elasto-capillary Bundling of Micropillar Arrays: A Direct Visualization.
Langmuir,
Vol. 36,
Issue. 39,
p.
11581.
Pepona, M.
Shek, A. C. M.
Semprebon, C.
Krüger, T.
and
Kusumaatmaja, H.
2021.
Modeling ternary fluids in contact with elastic membranes.
Physical Review E,
Vol. 103,
Issue. 2,
Sasaki, Yuta
Yamazaki, Tomoya
and
Kimura, Yuki
2022.
Liquid-Cell Transmission Electron Microscopy Observation of Two-Step Collapse Dynamics of Silicon Nanopillars on Evaporation of Propan-2-ol: Implications for Semiconductor Integration Density.
ACS Applied Nano Materials,
Vol. 5,
Issue. 7,
p.
9495.
 $N=O(10^4)$. A linear stability analysis shows that pairwise coalescence is always the most unstable mode of deformation. However, the numerical simulations show that the cluster sizes actually produced by coalescence from a small white-noise perturbation have a distribution that depends on the relative strength of surface tension and elasticity, as measured by an elastocapillary number
$N=O(10^4)$. A linear stability analysis shows that pairwise coalescence is always the most unstable mode of deformation. However, the numerical simulations show that the cluster sizes actually produced by coalescence from a small white-noise perturbation have a distribution that depends on the relative strength of surface tension and elasticity, as measured by an elastocapillary number 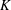 $K$. Both the maximum cluster size and the mean cluster size scale like
$K$. Both the maximum cluster size and the mean cluster size scale like 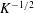 $K^{-1/2}$ for small
$K^{-1/2}$ for small  $K$. An analytical solution for the response of the system to a localized perturbation shows that such perturbations generate propagating disturbance fronts, which leave behind ‘frozen-in’ clusters of a predictable size that also depends on
$K$. An analytical solution for the response of the system to a localized perturbation shows that such perturbations generate propagating disturbance fronts, which leave behind ‘frozen-in’ clusters of a predictable size that also depends on 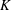 $K$. A good quantitative comparison between the cluster-size statistics from noisy perturbations and this ‘frozen-in’ cluster size suggests that propagating fronts may play a crucial role in the dynamics of coalescence.
$K$. A good quantitative comparison between the cluster-size statistics from noisy perturbations and this ‘frozen-in’ cluster size suggests that propagating fronts may play a crucial role in the dynamics of coalescence.