Article contents
Field-induced macroscopic flow of a dilute self-assembling magnetic colloid under rotating magnetic fields
Published online by Cambridge University Press: 19 February 2024
Abstract
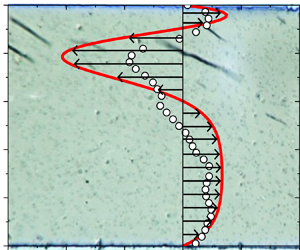
Flow generation by colloidal motors activated by external stimuli is an important issue for active matter physics and several nanotechnological or biomedical applications. For instance, flow recirculation generated by rotating magnetic self-assemblies allows effective ‘pumping’ of a thrombolytic drug towards a blood clot along a blocked vessel. However, the physics of the flow generation in this case remains still poorly explored. This study is focused on the generation of a recirculation flow of a magnetic colloid (aqueous suspension of iron oxide nanoparticles with partially screened electrostatic repulsion) within a closed microfluidic channel via application of an external rotating magnetic field. The colloid undergoes reversible phase separation manifested through the appearance of micron-sized elongated aggregates. They synchronously rotate with the magnetic field and can generate macroscopic flows only in the presence of gradients of the aggregate concentration across the channel induced by superposition of a weak magnetic field gradient to the homogeneous rotating field. We achieve recirculation flows with a characteristic speed  ${\sim} 5{-}8\;{\rm \mu}\textrm{m}\;{\textrm{s}^{ - \textrm{1}}}$ at low magnetic field amplitude and frequency (
${\sim} 5{-}8\;{\rm \mu}\textrm{m}\;{\textrm{s}^{ - \textrm{1}}}$ at low magnetic field amplitude and frequency ( ${H_0} \approx 3{-}10\;\textrm{kA}\;{\textrm{m}^{ - 1}}$,
${H_0} \approx 3{-}10\;\textrm{kA}\;{\textrm{m}^{ - 1}}$,  ${f = 5{-}15\ \textrm{Hz}}$) at low nanoparticle volume fraction
${f = 5{-}15\ \textrm{Hz}}$) at low nanoparticle volume fraction  ${\varphi _p} = (1.6{-}3.2) \times {10^{ - 3}}$. The concentration and velocity profiles have been assessed experimentally through particle tracking and particle image velocimetry, and have also been computed using the hydrodynamic diffusion approach coupled with the momentum balance equation with a magnetic torque term. The model correctly reproduces the shape of the experimental concentration and velocity fields and explains complex behaviours of the average recirculation speed as a function of governing parameters (
${\varphi _p} = (1.6{-}3.2) \times {10^{ - 3}}$. The concentration and velocity profiles have been assessed experimentally through particle tracking and particle image velocimetry, and have also been computed using the hydrodynamic diffusion approach coupled with the momentum balance equation with a magnetic torque term. The model correctly reproduces the shape of the experimental concentration and velocity fields and explains complex behaviours of the average recirculation speed as a function of governing parameters ( ${H_0}$, f,
${H_0}$, f,  ${\varphi _p}$, channel size).
${\varphi _p}$, channel size).
Information
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2024. Published by Cambridge University Press
References
- 1
- Cited by


