No CrossRef data available.
Article contents
Experimental study of axial flow evolution and area-varying waves in concentrated vortices
Published online by Cambridge University Press: 06 January 2023
Abstract
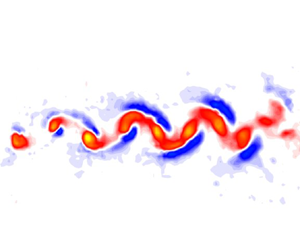
The dynamics of axial flow is investigated in initially two-dimensional (2-D) concentrated line vortices that are subsequently cut by a thin wall. The vortices are formed in the wake of an airfoil oscillating sinusoidally at small amplitude, and their circulation and peak vorticity are controlled by the choice of oscillation frequency. The flow field is quantified in terms of the spatio-temporal evolution of axial (spanwise) velocity using one-component molecular tagging velocimetry, and simultaneous measurements of spanwise vorticity and axial velocity at selected planes away from the cutting wall using stereoscopic molecular tagging velocimetry. Results show that the characteristics of the vortices cut by the wall are initially in good agreement with the baseline 2-D vortices. However, as the axial flow develops near the cutting wall and propagates away from it farther downstream, the structure of the initially 2-D Gaussian-shaped vortices quickly changes to a highly distorted region(s) of vorticity near the wall. Data over planes one and two core radii away from the wall reveal appreciable axial velocity both toward and away from the wall, whereas at locations farther away the axial velocity is primarily away from the wall. The data reveal the existence of small amplitude area-varying waves that propagate away from the wall along the vortex core. These disturbances are associated with local changes in the vortex axial velocity and peak vorticity. The propagation speed of these disturbances is found to agree with predictions using the analytical model of Lundgren & Ashurst (J. Fluid Mech., vol. 200, 1989, pp. 283–307).
JFM classification
Information
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2023. Published by Cambridge University Press


