Article contents
Experimental investigation of the three-dimensional flow structure around a pair of cubes immersed in the inner part of a turbulent channel flow
Published online by Cambridge University Press: 17 May 2021
Abstract
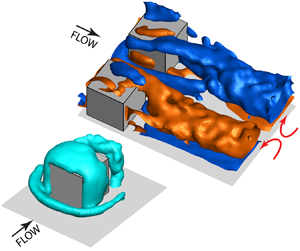
The origin and evolution of the three-dimensional flow structures around a pair of roughness cubes embedded in the inner part of a turbulent channel flow ( ${\textit{Re}}_{\tau \infty }=2300$, where
${\textit{Re}}_{\tau \infty }=2300$, where  ${\textit{Re}}_{\tau \infty}$ is the friction Reynolds number of the incoming turbulent channel flow) are measured using microscopic dual-view tomographic holography. The cubes’ height,
${\textit{Re}}_{\tau \infty}$ is the friction Reynolds number of the incoming turbulent channel flow) are measured using microscopic dual-view tomographic holography. The cubes’ height,  $a=1$ mm, corresponds to 91 wall units or 3.9 % of the half-channel height. They are aligned in the spanwise direction and separated by a, 1.5a and 2.5a. This paper focuses on the mean flow structure, and the data resolution allows detailed characterization of the open separated regions upstream, along the sides, on top of and behind the cubes, as well as measurements of wall shear stresses from velocity gradients. The flow features a horseshoe vortex, a vortical canopy engulfing each cube, a near wake arch-like vortex and multiple interacting streamwise vortices. Most of the boundary layer vorticity is entrained into the horseshoe vortex. The canopy, consisting of wall-normal vorticity to the sides, and spanwise vorticity on top of the cube, originates from the front surface. The streamwise vortices originate from realignment of the other components along the corners of the front surface. Merging of streamwise structures around and behind each cube causes formation of a large streamwise vortex rotating in the same direction as the inner horseshoe leg, with remnants of the outer leg under it. This merging occurs earlier and the entire flow structure becomes more asymmetric with decreasing spacing. Peaks and minima in the distributions of the wall shear stress are associated with the formation of and interactions among the near-wall vortices.
$a=1$ mm, corresponds to 91 wall units or 3.9 % of the half-channel height. They are aligned in the spanwise direction and separated by a, 1.5a and 2.5a. This paper focuses on the mean flow structure, and the data resolution allows detailed characterization of the open separated regions upstream, along the sides, on top of and behind the cubes, as well as measurements of wall shear stresses from velocity gradients. The flow features a horseshoe vortex, a vortical canopy engulfing each cube, a near wake arch-like vortex and multiple interacting streamwise vortices. Most of the boundary layer vorticity is entrained into the horseshoe vortex. The canopy, consisting of wall-normal vorticity to the sides, and spanwise vorticity on top of the cube, originates from the front surface. The streamwise vortices originate from realignment of the other components along the corners of the front surface. Merging of streamwise structures around and behind each cube causes formation of a large streamwise vortex rotating in the same direction as the inner horseshoe leg, with remnants of the outer leg under it. This merging occurs earlier and the entire flow structure becomes more asymmetric with decreasing spacing. Peaks and minima in the distributions of the wall shear stress are associated with the formation of and interactions among the near-wall vortices.
Information
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2021. Published by Cambridge University Press
References
REFERENCES
- 11
- Cited by


