Published online by Cambridge University Press: 23 January 2020
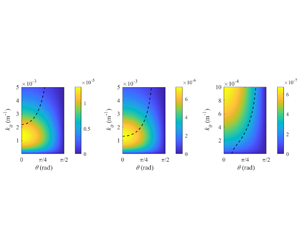
We consider interactions between surface and interfacial waves in a two-layer system. Our approach is based on the Hamiltonian structure of the equations of motion, and includes the general procedure for diagonalization of the quadratic part of the Hamiltonian. Such diagonalization allows us to derive the interaction cross-section between surface and interfacial waves and to derive the coupled kinetic equations describing spectral energy transfers in this system. Our kinetic equation allows resonant and near-resonant interactions. We find that the energy transfers are dominated by the class III resonances of Alam (J. Fluid Mech., vol. 691, 2012, pp. 267–278). We apply our formalism to calculate the rate of growth for interfacial waves for different values of wind velocity. Using our kinetic equation, we also consider the energy transfer from wind-generated surface waves to interfacial waves for the case when the spectrum of the surface waves is given by the JONSWAP spectrum and interfacial waves are initially absent. We find that such energy transfer can occur along a time scale of hours; there is a range of wind speeds for the most effective energy transfer at approximately the wind speed corresponding to white capping of the sea. Furthermore, interfacial waves oblique to the direction of the wind are also generated.