Article contents
Evolution, propagation and interactions with topography of hurricane-like vortices in a moist-convective rotating shallow-water model
Published online by Cambridge University Press: 10 September 2020
Abstract
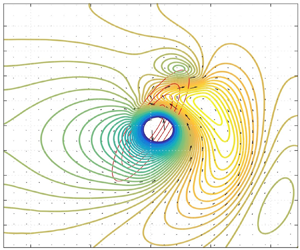
The so-called moist-convective shallow-water model, which incorporates moist convection in a simple albeit self-consistent way is used to analyse how intense localized vortices, with distributions of horizontal velocity and relative vorticity close to those observed in tropical cyclones (TC), evolve and interact with topography on the  $\beta$-plane at low latitudes. Instabilities of such TC-like vortices are studied first in the
$\beta$-plane at low latitudes. Instabilities of such TC-like vortices are studied first in the  $f$-plane approximation, and their development, interplay with beta-gyres and the role they play in vorticity redistribution and intensification are then analysed along the vortex trajectories on the
$f$-plane approximation, and their development, interplay with beta-gyres and the role they play in vorticity redistribution and intensification are then analysed along the vortex trajectories on the  $\beta$-plane, both in dry and moist-convective environments. Interactions of the vortices with an idealized topography in the form of zonal and meridional ridges and islands of elliptic form and the role of moist convection in these processes are then investigated, revealing rich vortex-dynamics patterns. The results can be helpful in crude analyses and predictions of the evolution of the barotropic component of TC, of their trajectories over the ocean and during landfall and of related condensation/precipitation patterns.
$\beta$-plane, both in dry and moist-convective environments. Interactions of the vortices with an idealized topography in the form of zonal and meridional ridges and islands of elliptic form and the role of moist convection in these processes are then investigated, revealing rich vortex-dynamics patterns. The results can be helpful in crude analyses and predictions of the evolution of the barotropic component of TC, of their trajectories over the ocean and during landfall and of related condensation/precipitation patterns.
Information
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2020. Published by Cambridge University Press
References
REFERENCES
- 14
- Cited by


