Article contents
Divergent Richtmyer–Meshkov instability on a heavy gas layer
Published online by Cambridge University Press: 24 March 2023
Abstract
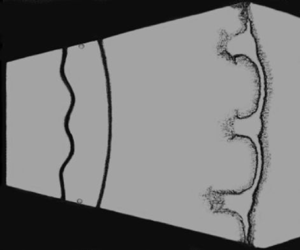
Experiments on divergent Richtmyer–Meshkov (RM) instability at a heavy gas layer are performed in a specially designed shock tube. A novel soap-film technique is extended to generate gas layers with controllable thicknesses and shapes. An unperturbed gas layer is first examined and its two interfaces are found to move uniformly at the early stage and be decelerated later. A general one-dimensional theory applicable to an arbitrary-thickness layer is established, which gives a good prediction of the layer motion in divergent geometry. Then, six kinds of perturbed SF $_6$ layers with various thicknesses and shapes surrounded by air are examined. At the early stage, the amplitude growths of the inner interface for various-thickness layers collapse quite well and also can be predicted by the Bell model for cylindrical RM instability at a single interface, which indicates a negligible interface coupling effect. Later, a rarefaction wave accelerates the inner interface, causing a dramatic rise in the growth rate. It is found that a thicker gas layer will result in a larger extent that the rarefaction wave can promote the instability growth. A modified Bell model accounting for both Rayleigh–Taylor (RT) instability and interface stretching caused by a rarefaction wave is established, which well reproduces the quick instability growth. At late stages, reverberating waves inside the layer are negligibly weak such that the inner interface growth is dominated by RM instability and RT stability. The major factors driving the outer interface development are a compression wave and interface coupling. A new interface coupling phenomenon existing uniquely in divergent geometry caused by the gradual thinning of the gas layer is observed and also modelled.
$_6$ layers with various thicknesses and shapes surrounded by air are examined. At the early stage, the amplitude growths of the inner interface for various-thickness layers collapse quite well and also can be predicted by the Bell model for cylindrical RM instability at a single interface, which indicates a negligible interface coupling effect. Later, a rarefaction wave accelerates the inner interface, causing a dramatic rise in the growth rate. It is found that a thicker gas layer will result in a larger extent that the rarefaction wave can promote the instability growth. A modified Bell model accounting for both Rayleigh–Taylor (RT) instability and interface stretching caused by a rarefaction wave is established, which well reproduces the quick instability growth. At late stages, reverberating waves inside the layer are negligibly weak such that the inner interface growth is dominated by RM instability and RT stability. The major factors driving the outer interface development are a compression wave and interface coupling. A new interface coupling phenomenon existing uniquely in divergent geometry caused by the gradual thinning of the gas layer is observed and also modelled.
JFM classification
Information
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2023. Published by Cambridge University Press
References
REFERENCES
- 10
- Cited by


