Article contents
Compressibility and variable inertia effects on heat transfer in turbulent impinging jets
Published online by Cambridge University Press: 28 January 2020
Abstract
Thisarticle shows the importance of flow compressibility on the heat transfer in confined impinging jets, and how it is driven by both the Mach number and the wall heat flux. Hence, we present a collection of cases at several Mach numbers with different heat-flux values applied at the impingement wall. The wall temperature scales linearly with the imposed heat flux and the adiabatic wall temperature is found to be purely governed by the flow compression. Especially for high heat-flux values, the non-constant wall temperature induces considerable differences in the thermal conductivity of the fluid. This phenomenon has to date not been discussed and it strongly modulates the Nusselt number. In contrast, the heat transfer coefficient is independent of the varying thermal properties of the fluid and the wall heat flux. Furthermore, we introduce the impingement efficiency, which highlights the areas of the wall where the temperature is influenced by compressibility effects. This parameter shows how the contribution of the flow compression to raising the wall temperature becomes more dominant as the heat flux decreases. Thus, knowing the adiabatic wall temperature is indispensable for obtaining the correct heat transfer coefficient when low heat-flux values are used, even at low Mach numbers. Lastly, a detailed analysis of the dilatation field also shows how the compressibility effects only affect the heat transfer in the vicinity of the stagnation point. These compressibility effects decay rapidly further away from the flow impingement, and the density changes along the developing boundary layer are caused instead by variable inertia effects.
Information
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2020. Published by Cambridge University Press
References
Otero-Pérez and Sandberg supplementary movie
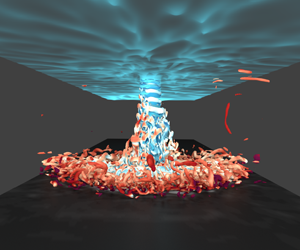
Animation of a confined turbulent impinging jet flow at $Re_D=10,000$ and $Ma=0.5$. The impingement wall--heated at a constant heat-flux--is coloured with instantaneous contours of the wall shear stress (brighter colours indicate higher $\tau_w$). The confinement wall imposes an isothermal condition with the same temperature as the jet flow. The lower temperature flow from the jet and along this confinement boundary is shown with light-blue coloured temperature isovolumes. The turbulent jet is represented with isosurfaces of Q coloured by temperature.
- 8
- Cited by

