Article contents
Complex-network analysis of high-frequency combustion instability in a model single-element rocket engine combustor
Published online by Cambridge University Press: 15 March 2023
Abstract
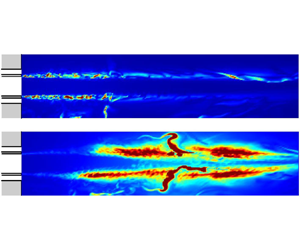
We study the spatio-temporal dynamics of high-frequency combustion instability in a model single-element rocket combustor using an acoustic energy flux-based spatial network. The acoustic energy source collapses by the formation of small communities with weak connection when the flame edge is attached to the injector rim. In contrast, large communities with strong connection are formed in the shear layer between the oxygen and hydrogen jets when the flame edge is detached from the injector rim, which has a significant impact on driving combustion instability. The switching between the attachment and detachment of the flame edge during combustion instability can be explained by the spectral-clustering-based transition network constructed from the pressure and flow velocity of the hydrogen jet at the injector exit, and the temperature near the injector rim.
JFM classification
Information
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2023. Published by Cambridge University Press
References
REFERENCES
- 7
- Cited by


