Published online by Cambridge University Press: 22 May 2019
In this two-part study, we present the development and analysis of a stochastic theory for characterizing the relative positions of monodisperse, low-inertia particle pairs that are settling rapidly in homogeneous isotropic turbulence. In the limits of small Stokes number and Froude number such that  $Fr\ll St_{\unicode[STIX]{x1D702}}\ll 1$, closures are developed for the drift and diffusion fluxes in the probability density function (p.d.f.) equation for the pair relative positions. The theory focuses on the relative motion of particle pairs in the dissipation regime of turbulence, i.e. for pair separations smaller than the Kolmogorov length scale. In this regime, the theory approximates the fluid velocity field in a reference frame following the primary particle as locally linear. In this part 1 paper, we present the derivation of closure approximations for the drift and diffusion fluxes in the p.d.f. equation for pair relative positions
$Fr\ll St_{\unicode[STIX]{x1D702}}\ll 1$, closures are developed for the drift and diffusion fluxes in the probability density function (p.d.f.) equation for the pair relative positions. The theory focuses on the relative motion of particle pairs in the dissipation regime of turbulence, i.e. for pair separations smaller than the Kolmogorov length scale. In this regime, the theory approximates the fluid velocity field in a reference frame following the primary particle as locally linear. In this part 1 paper, we present the derivation of closure approximations for the drift and diffusion fluxes in the p.d.f. equation for pair relative positions  $\boldsymbol{r}$. The drift flux contains the time integral of the third and fourth moments of the ‘seen’ fluid velocity gradients along the trajectories of primary particles. These moments may be analytically resolved by making approximations regarding the ‘seen’ velocity gradient. Accordingly, two closure forms are derived specifically for the drift flux. The first invokes the assumption that the fluid velocity gradient along particle trajectories has a Gaussian distribution. In the second drift closure, we account for the correlation time scales of dissipation rate and enstrophy by decomposing the velocity gradient into the strain-rate and rotation-rate tensors scaled by the turbulent dissipation rate and enstrophy, respectively. An analytical solution to the p.d.f.
$\boldsymbol{r}$. The drift flux contains the time integral of the third and fourth moments of the ‘seen’ fluid velocity gradients along the trajectories of primary particles. These moments may be analytically resolved by making approximations regarding the ‘seen’ velocity gradient. Accordingly, two closure forms are derived specifically for the drift flux. The first invokes the assumption that the fluid velocity gradient along particle trajectories has a Gaussian distribution. In the second drift closure, we account for the correlation time scales of dissipation rate and enstrophy by decomposing the velocity gradient into the strain-rate and rotation-rate tensors scaled by the turbulent dissipation rate and enstrophy, respectively. An analytical solution to the p.d.f.  $\langle P\rangle (r,\unicode[STIX]{x1D703})$ is then derived, where
$\langle P\rangle (r,\unicode[STIX]{x1D703})$ is then derived, where  $\unicode[STIX]{x1D703}$ is the spherical polar angle. It is seen that the p.d.f. has a power-law dependence on separation
$\unicode[STIX]{x1D703}$ is the spherical polar angle. It is seen that the p.d.f. has a power-law dependence on separation  $r$ of the form
$r$ of the form 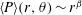 $\langle P\rangle (r,\unicode[STIX]{x1D703})\sim r^{\unicode[STIX]{x1D6FD}}$ with
$\langle P\rangle (r,\unicode[STIX]{x1D703})\sim r^{\unicode[STIX]{x1D6FD}}$ with 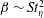 $\unicode[STIX]{x1D6FD}\sim St_{\unicode[STIX]{x1D702}}^{2}$ and
$\unicode[STIX]{x1D6FD}\sim St_{\unicode[STIX]{x1D702}}^{2}$ and  $\unicode[STIX]{x1D6FD}<0$, analogous to that for the radial distribution function of non-settling pairs. An explicit expression is derived for
$\unicode[STIX]{x1D6FD}<0$, analogous to that for the radial distribution function of non-settling pairs. An explicit expression is derived for 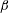 $\unicode[STIX]{x1D6FD}$ in terms of the drift and diffusion closures. The
$\unicode[STIX]{x1D6FD}$ in terms of the drift and diffusion closures. The  $\langle P\rangle (r,\unicode[STIX]{x1D703})$ solution also shows that, for a given
$\langle P\rangle (r,\unicode[STIX]{x1D703})$ solution also shows that, for a given  $r$, the clustering of
$r$, the clustering of 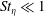 $St_{\unicode[STIX]{x1D702}}\ll 1$ particles is only weakly anisotropic, which is in conformity with prior observations from direct numerical simulations of isotropic turbulence containing settling particles.
$St_{\unicode[STIX]{x1D702}}\ll 1$ particles is only weakly anisotropic, which is in conformity with prior observations from direct numerical simulations of isotropic turbulence containing settling particles.
Present address: Department of Chemical Engineering, University of Missouri, Columbia, MO 65211, USA.
Please note that this article has a (1).