Article contents
Cavitation about a jet in crossflow
Published online by Cambridge University Press: 04 March 2015
Abstract
Cavitation occurrence about a jet in crossflow is investigated experimentally in a variable-pressure water tunnel using still and high-speed photography. The 0.012 m diameter jet is injected on the centreplane of a 0.6 m square test section at jet to freestream velocity ratios ranging from 0.2 to 1.6, corresponding to jet-velocity-based Reynolds numbers of 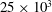 $25\times 10^{3}$ to
$25\times 10^{3}$ to  $160\times 10^{3}$ respectively. Measurements were made at a fixed freestream-based Reynolds number, for which the ratio of the undisturbed boundary layer thickness to jet diameter is 1.18. The cavitation number was varied from inception (up to about 10) down to 0.1. Inception is investigated acoustically for bounding cases of high and low susceptibility to phase change. The influence of velocity ratio and cavitation number on cavity topology and geometry are quantified from the photography. High-speed photographic recordings made at 6 kHz provide insight into cavity dynamics, and derived time series of spatially averaged pixel intensities enable frequency analysis of coherent phenomena. Cavitation inception was found to occur in the high-shear regions either side of the exiting jet and to be of an intermittent nature, increasing in occurrence and duration from 0 to 100 % probability with decreasing cavitation number or increasing jet to freestream velocity ratio. The frequency and duration of individual events strongly depends on the cavitation nuclei supply within the approaching boundary layer. Macroscopic cavitation develops downstream of the jet with reduction of the cavitation number beyond inception, the length of which has a power-law dependence on the cavitation number and a linear dependence on the jet to freestream velocity ratio. The cavity closure develops a re-entrant jet with increase in length forming a standing wave within the cavity. For sufficiently low cavitation numbers the projection of the re-entrant jet fluid no longer reaches the cavity leading edge, analogous to supercavitation forming about solid cavitators. Hairpin-shaped vortices are coherently shed from the cavity closure via mechanisms of shear-layer roll-up similar to those shed from protuberances and jets in crossflow in single-phase flows. These vortices are shed at an apparently constant frequency, independent of the jet to freestream velocity ratio but decreasing in frequency with reducing cavitation number and cavity volume growth. Highly coherent cavitating vortices form along the leading part of the cavity due to instability of the jet upstream shear layer for jet to freestream velocity ratios greater than about 0.8. These vortices are cancelled and condense as they approach the trailing edge in the shear layer of opposing vorticity associated with the cavity closure and the hairpin vortex formation. For lower velocity ratios, where there is decreased jet penetration, the jet upstream shear velocity gradient reverses and vortices of the opposite sense form, randomly modulated by boundary layer turbulence.
$160\times 10^{3}$ respectively. Measurements were made at a fixed freestream-based Reynolds number, for which the ratio of the undisturbed boundary layer thickness to jet diameter is 1.18. The cavitation number was varied from inception (up to about 10) down to 0.1. Inception is investigated acoustically for bounding cases of high and low susceptibility to phase change. The influence of velocity ratio and cavitation number on cavity topology and geometry are quantified from the photography. High-speed photographic recordings made at 6 kHz provide insight into cavity dynamics, and derived time series of spatially averaged pixel intensities enable frequency analysis of coherent phenomena. Cavitation inception was found to occur in the high-shear regions either side of the exiting jet and to be of an intermittent nature, increasing in occurrence and duration from 0 to 100 % probability with decreasing cavitation number or increasing jet to freestream velocity ratio. The frequency and duration of individual events strongly depends on the cavitation nuclei supply within the approaching boundary layer. Macroscopic cavitation develops downstream of the jet with reduction of the cavitation number beyond inception, the length of which has a power-law dependence on the cavitation number and a linear dependence on the jet to freestream velocity ratio. The cavity closure develops a re-entrant jet with increase in length forming a standing wave within the cavity. For sufficiently low cavitation numbers the projection of the re-entrant jet fluid no longer reaches the cavity leading edge, analogous to supercavitation forming about solid cavitators. Hairpin-shaped vortices are coherently shed from the cavity closure via mechanisms of shear-layer roll-up similar to those shed from protuberances and jets in crossflow in single-phase flows. These vortices are shed at an apparently constant frequency, independent of the jet to freestream velocity ratio but decreasing in frequency with reducing cavitation number and cavity volume growth. Highly coherent cavitating vortices form along the leading part of the cavity due to instability of the jet upstream shear layer for jet to freestream velocity ratios greater than about 0.8. These vortices are cancelled and condense as they approach the trailing edge in the shear layer of opposing vorticity associated with the cavity closure and the hairpin vortex formation. For lower velocity ratios, where there is decreased jet penetration, the jet upstream shear velocity gradient reverses and vortices of the opposite sense form, randomly modulated by boundary layer turbulence.
JFM classification
Information
- Type
- Papers
- Information
- Copyright
- © 2015 Cambridge University Press
References
Brandner et al. supplementary movie
High-speed movie of cavitation about jet with R = 1.2 and σ = 0.2 acquired at 6 kHz, played at 30 frames per second. Shear layer vortices are evident rolling in a clockwise direction (negative vorticity) before being canceled and reversed in the positive vorticity associated with the re-entrant jet type cavity closure and hairpin formation.
Brandner et al. supplementary movie
High-speed movie of cavitation about jet with R = 1.2 and σ = 0.2 acquired at 6 kHz, played at 30 frames per second. Shear layer vortices are evident rolling in a clockwise direction (negative vorticity) before being canceled and reversed in the positive vorticity associated with the re-entrant jet type cavity closure and hairpin formation.
Brandner et al. supplementary movie
High-speed movie of cavitation about jet with R = 0.8 and σ = 0.1 acquired at 6 kHz, played at 30 frames per second. The cavity is glassy and transparent toward the leading edge as opposed to the downstream region where the cavity surface remains opaque due to the breaking wave formed by the re-entrant jet inside the cavity.
Brandner et al. supplementary movie
High-speed movie of cavitation about jet with R = 0.8 and σ = 0.1 acquired at 6 kHz, played at 30 frames per second. The cavity is glassy and transparent toward the leading edge as opposed to the downstream region where the cavity surface remains opaque due to the breaking wave formed by the re-entrant jet inside the cavity.
Brandner et al. supplementary movie
High-speed movie of cavitation about jet with R = 0.6 and σ = 0.2 acquired at 6 kHz, played at 30 frames per second. Wave-like undulations can be seen on the cavity, typical for all R < 0.8, and have the oppositive sign (ie. positive vorticity) to the shear layer vortices evident for R > 0.8.
Brandner et al. supplementary movie
High-speed movie of cavitation about jet with R = 0.6 and σ = 0.2 acquired at 6 kHz, played at 30 frames per second. Wave-like undulations can be seen on the cavity, typical for all R < 0.8, and have the oppositive sign (ie. positive vorticity) to the shear layer vortices evident for R > 0.8.
Brandner et al. supplementary movie
High-speed movie of cavitation about jet with R = 0.6 and σ = 0.6 acquired at 6 kHz, played at 30 frames per second. The high cavitation number and low velocity ratio prevent development of visible shear layer vortices.
Brandner et al. supplementary movie
High-speed movie of cavitation about jet with R = 0.6 and σ = 0.6 acquired at 6 kHz, played at 30 frames per second. The high cavitation number and low velocity ratio prevent development of visible shear layer vortices.
Brandner et al. supplementary movie
High-speed movie of cavitation about jet with R = 1.6 and σ = 0.2 acquired at 6 kHz, played at 30 frames per second. The large cavity volume causes pairing and/or merging of shear layer vortices.
Brandner et al. supplementary movie
High-speed movie of cavitation about jet with R = 1.6 and σ = 0.2 acquired at 6 kHz, played at 30 frames per second. The large cavity volume causes pairing and/or merging of shear layer vortices.
Brandner et al. supplementary movie
High-speed movie of cavitation about jet with R = 1.2 and σ = 0.6 acquired at 6 kHz, played at 30 frames per second. The cavity length is similar to the wavelength of the shear layer vortices, limiting their development before they are canceled by the counter-rotating cavity closure.
Brandner et al. supplementary movie
High-speed movie of cavitation about jet with R = 1.2 and σ = 0.6 acquired at 6 kHz, played at 30 frames per second. The cavity length is similar to the wavelength of the shear layer vortices, limiting their development before they are canceled by the counter-rotating cavity closure.
- 23
- Cited by


