Article contents
Buoyancy scale effects in large-eddy simulations of stratified turbulence
Published online by Cambridge University Press: 30 July 2014
Abstract
In this paper large-eddy simulations (LES) of forced stratified turbulence using two common subgrid scale (SGS) models, the Kraichnan and Smagorinsky models, are studied. As found in previous studies using regular and hyper-viscosity, vorticity contours show elongated horizontal motions, which are layered in the vertical direction, along with intermittent Kelvin–Helmholtz (KH) instabilities. Increased stratification causes the layer thickness to collapse towards the dissipation scale, ultimately suppressing these instabilities. The vertical energy spectra are relatively flat out to a local maximum, which varies with the buoyancy frequency  $\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}N$ . The horizontal energy spectra depend on the grid spacing
$\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}N$ . The horizontal energy spectra depend on the grid spacing 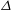 $\varDelta $ ; if the resolution is fine enough, the horizontal spectrum shows an approximately
$\varDelta $ ; if the resolution is fine enough, the horizontal spectrum shows an approximately 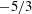 $-5/3$ slope along with a bump at the buoyancy wavenumber
$-5/3$ slope along with a bump at the buoyancy wavenumber 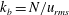 $k_b = N/u_{rms}$ , where
$k_b = N/u_{rms}$ , where 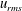 $u_{rms}$ is the root-mean-square (r.m.s.) velocity. Our results show that there is a critical value of the grid spacing
$u_{rms}$ is the root-mean-square (r.m.s.) velocity. Our results show that there is a critical value of the grid spacing  $\varDelta $ , below which dynamics of stratified turbulence are well-captured in LES. This critical
$\varDelta $ , below which dynamics of stratified turbulence are well-captured in LES. This critical 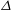 $\varDelta $ depends on the buoyancy scale
$\varDelta $ depends on the buoyancy scale 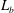 $L_b$ and varies with different SGS models: the Kraichnan model requires
$L_b$ and varies with different SGS models: the Kraichnan model requires 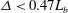 $\varDelta < 0.47 L_b$ , while the Smagorinsky model requires
$\varDelta < 0.47 L_b$ , while the Smagorinsky model requires 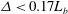 $\varDelta < 0.17 L_b$ . In other words, the Smagorinsky model is significantly more costly than the Kraichnan approach, as it requires three times the resolution to adequately capture stratified turbulence.
$\varDelta < 0.17 L_b$ . In other words, the Smagorinsky model is significantly more costly than the Kraichnan approach, as it requires three times the resolution to adequately capture stratified turbulence.
Information
- Type
- Papers
- Information
- Copyright
- © 2014 Cambridge University Press
References
- 30
- Cited by

