Article contents
Boundary conditions for free surface inlet and outlet problems
Published online by Cambridge University Press: 10 August 2012
Abstract
We investigate and compare the boundary conditions that are to be applied to free-surface problems involving inlet and outlets of Newtonian fluid, typically found in coating processes. The flux of fluid is a priori known at an inlet, but unknown at an outlet, where it is governed by the local behaviour near the film-forming meniscus. In the limit of vanishing capillary number  it is well known that the flux scales with
it is well known that the flux scales with  , but this classical result is non-uniform as the contact angle approaches
, but this classical result is non-uniform as the contact angle approaches 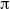 . By examining this limit we find a solution that is uniformly valid for all contact angles. Furthermore, by considering the far-field behaviour of the free surface we show that there exists a critical capillary number above which the problem at an inlet becomes over-determined. The implications of this result for the modelling of coating flows are discussed.
. By examining this limit we find a solution that is uniformly valid for all contact angles. Furthermore, by considering the far-field behaviour of the free surface we show that there exists a critical capillary number above which the problem at an inlet becomes over-determined. The implications of this result for the modelling of coating flows are discussed.
Information
- Type
- Papers
- Information
- Copyright
- Copyright © Cambridge University Press 2012
References
- 10
- Cited by

