Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Zhang, Kangping
Sandham, Neil
and
Hu, Zhiwei
2012.
Numerical Simulations of Global Instability In Separated Flows At High Mach Number.
Melo De Sousa, Joao
and
Camara, Jorge
2013.
Numerical Study on the Use of a Sinusoidal Leading Edge for Passive Stall Control at Low Reynolds Number.
Cambonie, Tristan
and
Aider, Jean-Luc
2014.
Transition scenario of the round jet in crossflow topology at low velocity ratios.
Physics of Fluids,
Vol. 26,
Issue. 8,
Peplinski, A.
Schlatter, P.
Fischer, P. F.
and
Henningson, D. S.
2014.
Spectral and High Order Methods for Partial Differential Equations - ICOSAHOM 2012.
Vol. 95,
Issue. ,
p.
349.
Sakai, E.
Takahashi, T.
and
Watanabe, H.
2014.
Large-eddy simulation of an inclined round jet issuing into a crossflow.
International Journal of Heat and Mass Transfer,
Vol. 69,
Issue. ,
p.
300.
Cerqueira, Stéphane
and
Sipp, Denis
2014.
Eigenvalue sensitivity, singular values and discrete frequency selection mechanism in noise amplifiers: the case of flow induced by radial wall injection.
Journal of Fluid Mechanics,
Vol. 757,
Issue. ,
p.
770.
Carini, M.
Giannetti, F.
and
Auteri, F.
2014.
On the origin of the flip–flop instability of two side-by-side cylinder wakes.
Journal of Fluid Mechanics,
Vol. 742,
Issue. ,
p.
552.
Bobinski, T.
Goujon-Durand, S.
and
Wesfreid, J. E.
2014.
Instabilities in the wake of a circular disk.
Physical Review E,
Vol. 89,
Issue. 5,
Loiseau, Jean-Christophe
Robinet, Jean-Christophe
Cherubini, Stefania
and
Leriche, Emmanuel
2014.
Investigation of the roughness-induced transition: global stability analyses and direct numerical simulations.
Journal of Fluid Mechanics,
Vol. 760,
Issue. ,
p.
175.
Peplinski, A.
Schlatter, P.
and
Henningson, D.S.
2015.
Global stability and optimal perturbation for a jet in cross-flow.
European Journal of Mechanics - B/Fluids,
Vol. 49,
Issue. ,
p.
438.
Bistrian, D. A.
and
Navon, I. M.
2015.
An improved algorithm for the shallow water equations model reduction: Dynamic Mode Decomposition vs POD.
International Journal for Numerical Methods in Fluids,
Vol. 78,
Issue. 9,
p.
552.
Brandner, P. A.
Pearce, B. W.
and
de Graaf, K. L.
2015.
Cavitation about a jet in crossflow.
Journal of Fluid Mechanics,
Vol. 768,
Issue. ,
p.
141.
Marquet, O.
and
Larsson, M.
2015.
Global wake instabilities of low aspect-ratio flat-plates.
European Journal of Mechanics - B/Fluids,
Vol. 49,
Issue. ,
p.
400.
Camarri, Simone
2015.
Flow control design inspired by linear stability analysis.
Acta Mechanica,
Vol. 226,
Issue. 4,
p.
979.
Alvergue, Luis
Babaee, Hessam
Gu, Guoxiang
and
Acharya, Sumanta
2015.
Feedback Stabilization of a Reduced-Order Model of a Jet in Crossflow.
AIAA Journal,
Vol. 53,
Issue. 9,
p.
2472.
Keller, Michael A.
and
Kloker, Markus J.
2015.
Effusion Cooling and Flow Tripping in Laminar Supersonic Boundary-Layer Flow.
AIAA Journal,
Vol. 53,
Issue. 4,
p.
902.
Peplinski, A.
Schlatter, P.
and
Henningson, D. S.
2015.
Instability and Control of Massively Separated Flows.
Vol. 107,
Issue. ,
p.
7.
Peplinski, A.
Schlatter, P.
and
Henningson, D. S.
2015.
Direct and Large-Eddy Simulation IX.
Vol. 20,
Issue. ,
p.
207.
Zhou, Wenwu
and
Hu, Hui
2016.
Improvements of film cooling effectiveness by using Barchan dune shaped ramps.
International Journal of Heat and Mass Transfer,
Vol. 103,
Issue. ,
p.
443.
Li, Guoneng
Zheng, Youqu
Yang, Huawen
Guo, Wenwen
and
Xu, Yousheng
2016.
Lattice Boltzmann simulation of a laminar square jet in cross flows.
Chinese Journal of Chemical Engineering,
Vol. 24,
Issue. 11,
p.
1505.
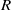 . We observe that, as the ratio
. We observe that, as the ratio 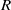 increases, the flow evolves from simple periodic vortex shedding (a limit cycle) to more complicated quasi-periodic behaviour, before finally becoming turbulent, as seen in the simulation of Bagheri et al. (J. Fluid. Mech., vol. 624, 2009b, pp. 33–44). The value of
increases, the flow evolves from simple periodic vortex shedding (a limit cycle) to more complicated quasi-periodic behaviour, before finally becoming turbulent, as seen in the simulation of Bagheri et al. (J. Fluid. Mech., vol. 624, 2009b, pp. 33–44). The value of  at which the first bifurcation occurs for our numerical set-up is found, and shedding of hairpin vortices characteristic of a shear layer instability is observed. We focus on this first bifurcation, and find that a global linear stability analysis predicts well the frequency and initial growth rate of the nonlinear DNS at the critical value of
at which the first bifurcation occurs for our numerical set-up is found, and shedding of hairpin vortices characteristic of a shear layer instability is observed. We focus on this first bifurcation, and find that a global linear stability analysis predicts well the frequency and initial growth rate of the nonlinear DNS at the critical value of 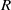 and that good qualitative predictions about the dynamics can still be made at slightly higher values of
and that good qualitative predictions about the dynamics can still be made at slightly higher values of  where multiple unstable eigenmodes are present. In addition, we compute the adjoint global eigenmodes, and find that the overlap of the direct and the adjoint eigenmode, also known as a ‘wavemaker’, provides evidence that the source of the first instability lies in the shear layer just downstream of the jet.
where multiple unstable eigenmodes are present. In addition, we compute the adjoint global eigenmodes, and find that the overlap of the direct and the adjoint eigenmode, also known as a ‘wavemaker’, provides evidence that the source of the first instability lies in the shear layer just downstream of the jet.
