Article contents
Barotropic to baroclinic energy conversion using a time-varying background density
Published online by Cambridge University Press: 11 May 2021
Abstract
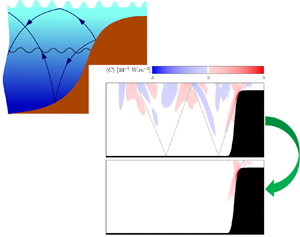
Internal wave generation is fundamentally the conversion of barotropic to baroclinic energy that often occurs due to vertical acceleration of stratified flows over topographic features. Acceleration results in a phase lag between density (pressure) perturbations and the barotropic velocity. To estimate the conversion of barotropic to baroclinic energy, the density perturbation is often calculated using a time-invariant background density. Other phenomena, however, can also alter the phasing of density perturbations and vertical velocities, such as barotropic tidal heaving and internal wave interactions. Consequently, accurately accounting for these dynamics in energy budgets is important. Tidal averaging or modal decomposition are often used to isolate topographic energy conversion in the presence of these other phenomena. However, while effective, these methods do not provide insights into the dynamics of conversion either through time or over depth. Here, we present a new analytical approach to calculating barotropic to baroclinic conversion using a time-varying background density. Our method results in an additional term in the baroclinic energy budget that directly accounts for barotropic tidal heaving and internal wave interactions, depending on the formulation of the background density. The tidally averaged, domain-integrated conversion rate is consistent across methods. Isolation of topographic conversion demonstrates that conversion due to interactions between internal wave beams and barotropic tidal heaving lead to relatively small differences in the overall conversion. However, using a time-varying background density allows for full decomposition of barotropic to baroclinic conversion through time and the identification of regions where negative conversion related to mixing actually occurs.
Information
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2021. Published by Cambridge University Press
References
REFERENCES
- 2
- Cited by


