Published online by Cambridge University Press: 24 September 2021
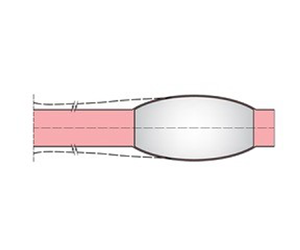
A mechanical theory is described for a phenomenon in the surgical procedure of resuscitative endovascular balloon occlusion of the aorta (REBOA). In this procedure a balloon is pushed into the aorta by a catheter and then inflated in order to stop haemorrhage. One of the hazards of this procedure is the tendency for the balloon to migrate away from its intended position. This work examines the mechanics of balloon anchoring and migration by analysing the effects of pressure waves, the sheet flow and solid friction in the thin gap between the walls of the aorta and balloon. A viscoelastic model is adopted for the aorta wall for pressure waves between the left ventricle and the balloon. The lubrication approximation is used for blood flow in the thin gap between the walls of the balloon and aorta. Samples of quantitative predictions are discussed on how the inflation pressure and balloon characteristics affect the balloon anchoring and migration. The crucial roles of solid friction and balloon placement are pointed out, which should help in guiding the manufacturing of balloons and their usage in the field.