Article contents
Wake transitions behind a cube at low and moderate Reynolds numbers
Published online by Cambridge University Press: 01 June 2021
Abstract
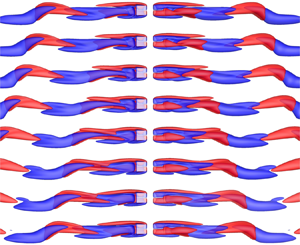
Wake transitions behind an isolated cube are investigated numerically over a range of Reynolds number ( ${{\textit {Re}}}$) 1–400. Four flow regimes, namely the orthogonal symmetry–steady (
${{\textit {Re}}}$) 1–400. Four flow regimes, namely the orthogonal symmetry–steady ( $1 \le {{\textit {Re}}} \le 205$), planar symmetry–steady (PSS,
$1 \le {{\textit {Re}}} \le 205$), planar symmetry–steady (PSS,  $210 \le {{\textit {Re}}} \le 250$), hairpin-vortex shedding (HS,
$210 \le {{\textit {Re}}} \le 250$), hairpin-vortex shedding (HS,  $255 \le {{\textit {Re}}} \le 305$) and chaotic vortex shedding (
$255 \le {{\textit {Re}}} \le 305$) and chaotic vortex shedding ( ${{\textit {Re}}} \ge 310$) regimes, are identified according to spatial symmetries and temporal development of the flow. A weakly nonlinear stability analysis, through a coupled pitchfork bifurcation model proposed in the present study, reveals that the bifurcation to the PSS regime is supercritical and the planar symmetry is retained in the PSS regime because stable points (four) exist only on the two symmetry planes perpendicular to the side faces of the cube. The supercritical Hopf bifurcation to the HS regime is induced by the excessive accumulation of circulation in the downstream tips of merged streamwise vortex tubes behind the cube that subsequently attract the vortex tubes of opposite signs to cut the tips off from them, leading to alternate shedding of streamwise vortex tubes. The planar symmetry observed in the PSS regime is preserved in the three vortex shedding states identified in the HS regime, i.e. single-frequency shedding (
${{\textit {Re}}} \ge 310$) regimes, are identified according to spatial symmetries and temporal development of the flow. A weakly nonlinear stability analysis, through a coupled pitchfork bifurcation model proposed in the present study, reveals that the bifurcation to the PSS regime is supercritical and the planar symmetry is retained in the PSS regime because stable points (four) exist only on the two symmetry planes perpendicular to the side faces of the cube. The supercritical Hopf bifurcation to the HS regime is induced by the excessive accumulation of circulation in the downstream tips of merged streamwise vortex tubes behind the cube that subsequently attract the vortex tubes of opposite signs to cut the tips off from them, leading to alternate shedding of streamwise vortex tubes. The planar symmetry observed in the PSS regime is preserved in the three vortex shedding states identified in the HS regime, i.e. single-frequency shedding ( $255 \le {{\textit {Re}}} \le 280$), quasi-periodic shedding (
$255 \le {{\textit {Re}}} \le 280$), quasi-periodic shedding ( $282 \le {{\textit {Re}}} \le 285$) and the high-order synchronised shedding (HS2,
$282 \le {{\textit {Re}}} \le 285$) and the high-order synchronised shedding (HS2,  $289 \le {{\textit {Re}}} \le 305$). A cascade of period doubling and period halving is discovered in the HS2 state, prior to the flow bifurcation to the chaotic vortex shedding regime, where the planar symmetry is broken. The critical
$289 \le {{\textit {Re}}} \le 305$). A cascade of period doubling and period halving is discovered in the HS2 state, prior to the flow bifurcation to the chaotic vortex shedding regime, where the planar symmetry is broken. The critical  ${{\textit {Re}}}$ values for the bifurcations to PSS and HS regimes are estimated to be
${{\textit {Re}}}$ values for the bifurcations to PSS and HS regimes are estimated to be  ${{\textit {Re}}} \approx 207.0$ and 252.0, respectively, through linear interpolation of the growth rate of flow instabilities.
${{\textit {Re}}} \approx 207.0$ and 252.0, respectively, through linear interpolation of the growth rate of flow instabilities.
JFM classification
Information
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2021. Published by Cambridge University Press
References
REFERENCES
- 14
- Cited by


