Article contents
Vorticity transport in a turbulent channel flow subjected to streamwise travelling waves
Published online by Cambridge University Press: 13 July 2023
Abstract
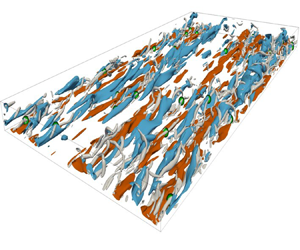
Direct numerical simulations of turbulent channel flow subjected to spanwise wall oscillations in the form of streamwise travelling waves (STW) were performed in an effort to elucidate the mechanism responsible for the observed drag reduction. We imposed large amplitudes to identify the proper effects of STW, while keeping the angular frequency and wavenumber fixed at a particular values. We primarily focus on the vorticity transport mechanism, to better understand the influence of STW actuation on the near-wall turbulence. We identify key terms appearing in the turbulent enstrophy transport equations that are directly linked to the STW actuation. The analysis reveals that the primary effect of the STW forcing is to attenuate the spanwise turbulent enstrophy at the wall, which is linked to the fluctuating wall shear stress. The suppression of the wall-normal turbulent enstrophy is deemed to be subordinate. To strengthen this point, we performed numerical experiments, where the streamwise fluctuating velocity, and consequently the spanwise vorticity, is artificially suppressed next to the wall. The anisotropic invariant maps show striking resemblance for large amplitude STW actuation and artificially forced cases. Detailed analysis of various structural features is provided, which includes the response of the near-wall streaks and shear layers of spanwise fluctuating velocity field. The quasistreamwise vortices, which play a key role in the regeneration mechanism, are shown to be pushed away from the wall, resulting in their weakened signature at the wall.
JFM classification
Information
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2023. Published by Cambridge University Press
References
- 4
- Cited by


