Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Goparaju, Hemanth
and
Gaitonde, Datta V.
2021.
Transition to turbulence on hypersonic flat plates induced by stochastic forcing.
Liu, Xuliang
Luo, Yong
Zhang, Shuhai
Li, Hu
Fan, Zhaolin
and
Sun, Xiaofeng
2021.
Direct Computation of Sound Generation by the Symmetric Vortex Merger.
p.
213.
Wang, Yimin
Luo, Yong
Li, Hu
and
Zhang, Shuhai
2021.
Numerical Study of Acoustic Wave Passing through the Temporal Mixing Layer.
p.
667.
Mao, Feng
Kang, Linlin
Liu, Luoqin
and
Wu, Jiezhi
2022.
A unified theory for gas dynamics and aeroacoustics in viscous compressible flows. Part I. Unbounded fluid.
Acta Mechanica Sinica,
Vol. 38,
Issue. 7,
Hu, Ya-Sen
Wan, Zhen-Hua
Ye, Chuang-Chao
Sun, De-Jun
and
Lu, Xi-Yun
2022.
Noise reduction mechanisms for insert-type serrations of the NACA-0012 airfoil.
Journal of Fluid Mechanics,
Vol. 941,
Issue. ,
Mao, Feng
Liu, Luoqin
Kang, Linlin
Wu, Jiezhi
Zhang, Pengjunyi
and
Wan, Zhenhua
2022.
A unified theory for gas dynamics and aeroacoustics in viscous compressible flows. Part II. Sources on solid boundary.
Acta Mechanica Sinica,
Vol. 38,
Issue. 12,
Hu, Ya-Sen
Zhang, Peng-Jun-Yi
Wan, Zhen-Hua
Liu, Nan-Sheng
Sun, De-Jun
and
Lu, Xi-Yun
2022.
Effects of trailing-edge serration shape on airfoil noise reduction with zero incidence angle.
Physics of Fluids,
Vol. 34,
Issue. 10,
Liang, Long-Long
Wan, Zhen-Hua
Ye, Chuang-Chao
Zhang, Peng-Jun-Yi
Sun, De-Jun
and
Lu, Xi-Yun
2023.
Flow dynamics and noise generation mechanisms in supersonic underexpanded rectangular and planar jets.
AIP Advances,
Vol. 13,
Issue. 6,
Zhou, Zhiteng
Liu, Yi
Wang, Hongping
and
Wang, Shizhao
2023.
Mass-Conserved Solution to the Ffowcs-Williams and Hawkings Equation for Compact Source Regions.
Aerospace,
Vol. 10,
Issue. 2,
p.
148.
Tong, Yunjin
Xiong, Shiying
He, Xingzhe
Yang, Shuqi
Wang, Zhecheng
Tao, Rui
Liu, Runze
and
Zhu, Bo
2024.
RoeNet: Predicting discontinuity of hyperbolic systems from continuous data.
International Journal for Numerical Methods in Engineering,
Vol. 125,
Issue. 6,
Gao, An-Kang
Chen, Zhe
Liu, Luoqin
and
Lu, Xi-Yun
2024.
Lift enhancement for airfoil at high angle of attack using flow-induced vibrations.
Physics of Fluids,
Vol. 36,
Issue. 12,
Yu, Ming
Dong, SiWei
Yuan, XianXu
and
Xu, ChunXiao
2024.
Statistics and dynamics of coherent structures in compressible wall-bounded turbulence.
Science China Physics, Mechanics & Astronomy,
Vol. 67,
Issue. 12,
Chen, Tao
Wu, Jie-Zhi
Liu, Tianshu
and
Salazar, David M.
2024.
Boundary sources of velocity gradient tensor and its invariants.
Physics of Fluids,
Vol. 36,
Issue. 11,
Yu, Fu-Yang
Wan, Zhen-Hua
Hu, Ya-Sen
Sun, De-Jun
and
Lu, Xi-Yun
2024.
Analysis of wavy leading-edge noise reduction and source mechanism in rod-airfoil interactions.
Physics of Fluids,
Vol. 36,
Issue. 4,
Yu, Fuyang
Wan, Zhenhua
Hu, Yasen
and
Sun, Dejun
2025.
Effects of the wavy leading-edge wavelength on the reduction in the noise of the rod-airfoil configuration.
JUSTC,
Vol. 55,
Issue. 2,
p.
0202.
Zhao, Yu-Han
Ding, Jue
Weng, Pei-Fen
Zhou, Quan
Dong, Yu-Hong
and
Yang, Xiao-Quan
2025.
Method of aerodynamic noise source identification for cylinder flows.
Journal of Fluid Mechanics,
Vol. 1012,
Issue. ,
Yu, Fu-Yang
Wan, Zhen-Hua
Hu, Ya-Sen
Sun, De-Jun
and
Lu, Xi-Yun
2025.
Effects of leading-edge serration shape on noise reduction in rod-airfoil interactions.
The Journal of the Acoustical Society of America,
Vol. 157,
Issue. 1,
p.
215.
Liu, Hanfeng
Yan, Jia
Chen, Binnian
Tan, Zhaoguang
and
Yang, Xiaoquan
2025.
Numerical simulation of aerodynamic noise characteristics of square-wave cylinder at Reynolds number 3900.
Physics of Fluids,
Vol. 37,
Issue. 1,
Tighezza, Ammar M.
Nazir, Abrar
Khera, Ejaz Ahmad
Manzoor, Mumtaz
Ishfaq, Mudassir
and
Sharma, Ramesh
2025.
A DFT Approach to Study the Vacancy Ordered lead free Double Inorganic Perovskites Na2PtX6 (X = Cl, Br) for Sustainable Technologies.
Journal of Inorganic and Organometallic Polymers and Materials,
Vol. 35,
Issue. 2,
p.
1201.
 $D/Dt$ rather than the local time-rate operator
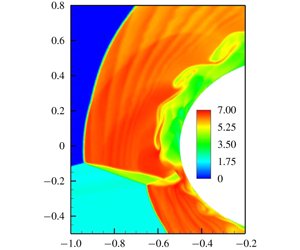
$D/Dt$ rather than the local time-rate operator  $\unicode[STIX]{x2202}/\unicode[STIX]{x2202}t$, and describes the sound mode by inhomogeneous advective wave equations. The sources of sound physical production inside the fluid are then examined at two levels. For the conventional formulation in terms of thermodynamic variables at the first level, we show that the universal kinematic source can be condensed to a scalar invariant of the surface deformation tensor. Further, in the formulation in terms of dilatation at the second level, we find that the sound mode in viscous and heat-conducting flow has sources from rich nonlinear couplings of vorticity, entropy and surface deformation, which cannot be disclosed at the first level. Preliminary numerical demonstration of the theoretical findings is made for two typical compressible flows, i.e. the interaction of two corotating Gaussian vortices and the unsteady type IV shock/shock interaction. The results obtained in this study provide a new theoretical basis for, and physical insight into, understanding various nonlinear longitudinal processes and the interactions therein.
$\unicode[STIX]{x2202}/\unicode[STIX]{x2202}t$, and describes the sound mode by inhomogeneous advective wave equations. The sources of sound physical production inside the fluid are then examined at two levels. For the conventional formulation in terms of thermodynamic variables at the first level, we show that the universal kinematic source can be condensed to a scalar invariant of the surface deformation tensor. Further, in the formulation in terms of dilatation at the second level, we find that the sound mode in viscous and heat-conducting flow has sources from rich nonlinear couplings of vorticity, entropy and surface deformation, which cannot be disclosed at the first level. Preliminary numerical demonstration of the theoretical findings is made for two typical compressible flows, i.e. the interaction of two corotating Gaussian vortices and the unsteady type IV shock/shock interaction. The results obtained in this study provide a new theoretical basis for, and physical insight into, understanding various nonlinear longitudinal processes and the interactions therein.