Article contents
Solute dispersion in pulsatile Casson fluid flow in a tube with wall absorption
Published online by Cambridge University Press: 23 March 2016
Abstract
The analysis of axial dispersion of solute is presented in a pulsatile flow of Casson fluid through a tube in the presence of interfacial mass transport due to irreversible first-order reaction catalysed by the tube wall. The theory of dispersion is studied by employing the generalized dispersion model proposed by Sankarasubramanian & Gill (Proc. R. Soc. Lond. A, vol. 333 (1592), 1973, pp. 115–132). This dispersion model describes the whole dispersion process in terms of three effective transport coefficients, i.e. exchange, convection and dispersion coefficients. In the present study, the effects of yield stress of Casson fluid 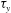 ${\it\tau}_{y}$ , wall absorption parameter
${\it\tau}_{y}$ , wall absorption parameter  ${\it\beta}$ , amplitude of fluctuating pressure component
${\it\beta}$ , amplitude of fluctuating pressure component  $e$ and Womersley frequency parameter
$e$ and Womersley frequency parameter  ${\it\alpha}$ on the dispersion process are discussed under the influence of pulsatile pressure gradient. In a pulsatile flow, the plug flow radius changes during the period of oscillation and it has an effect on the dispersion process. Even with the Casson fluid model also, in an oscillatory flow, for small values of
${\it\alpha}$ on the dispersion process are discussed under the influence of pulsatile pressure gradient. In a pulsatile flow, the plug flow radius changes during the period of oscillation and it has an effect on the dispersion process. Even with the Casson fluid model also, in an oscillatory flow, for small values of  ${\it\alpha}$ , the dispersion coefficient
${\it\alpha}$ , the dispersion coefficient 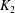 $K_{2}$ is positive, but when the value of
$K_{2}$ is positive, but when the value of  ${\it\alpha}$ is as large as 3,
${\it\alpha}$ is as large as 3,  $K_{2}$ takes both positive and negative values due to the fluctuations in the velocity profiles. This nature becomes more predominant for
$K_{2}$ takes both positive and negative values due to the fluctuations in the velocity profiles. This nature becomes more predominant for 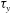 ${\it\tau}_{y}$ ,
${\it\tau}_{y}$ ,  $e$ and
$e$ and 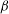 ${\it\beta}$ . It is observed that initially, for small time, the amplitude and magnitude of fluctuations of
${\it\beta}$ . It is observed that initially, for small time, the amplitude and magnitude of fluctuations of 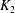 $K_{2}$ becomes more rapid and increases with time but it decreases after certain time and reaches a non-transient state for large time. Like in the case of Newtonian model, double frequency period for
$K_{2}$ becomes more rapid and increases with time but it decreases after certain time and reaches a non-transient state for large time. Like in the case of Newtonian model, double frequency period for 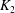 $K_{2}$ is observed at small time for large values of
$K_{2}$ is observed at small time for large values of  ${\it\alpha}$ with the Casson model for blood. It is seen that critical time for which
${\it\alpha}$ with the Casson model for blood. It is seen that critical time for which 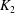 $K_{2}$ reaches a non-transient state is independent of
$K_{2}$ reaches a non-transient state is independent of 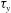 ${\it\tau}_{y}$ and
${\it\tau}_{y}$ and  $e$ but is dependent on
$e$ but is dependent on  ${\it\alpha}$ . It is also observed that the axial distribution of mean concentration
${\it\alpha}$ . It is also observed that the axial distribution of mean concentration 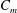 $C_{m}$ of solute depends on
$C_{m}$ of solute depends on  ${\it\tau}_{y}$ and
${\it\tau}_{y}$ and 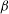 ${\it\beta}$ . But the effect of
${\it\beta}$ . But the effect of  $e$ and
$e$ and  ${\it\alpha}$ on
${\it\alpha}$ on 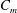 $C_{m}$ is not very significant. This dispersion model in non-Newtonian pulsatile flow can be applied to study the dispersion process in the cardiovascular system and blood oxygenators.
$C_{m}$ is not very significant. This dispersion model in non-Newtonian pulsatile flow can be applied to study the dispersion process in the cardiovascular system and blood oxygenators.
JFM classification
Information
- Type
- Papers
- Information
- Copyright
- © 2016 Cambridge University Press
References
- 75
- Cited by


