Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Chen, Sheng
Liu, Wenwei
and
Li, Shuiqing
2019.
A fast adhesive discrete element method for random packings of fine particles.
Chemical Engineering Science,
Vol. 193,
Issue. ,
p.
336.
Liu, Wenwei
and
Wu, Chuan-Yu
2020.
Modelling Complex Particle–Fluid Flow with a Discrete Element Method Coupled with Lattice Boltzmann Methods (DEM-LBM).
ChemEngineering,
Vol. 4,
Issue. 4,
p.
55.
Chen, Hongsheng
Liu, Wenwei
Chen, Zhiwei
and
Zheng, Zhong
2021.
A numerical study on the sedimentation of adhesive particles in viscous fluids using LBM-LES-DEM.
Powder Technology,
Vol. 391,
Issue. ,
p.
467.
Fu, Jianhong
Chen, Sheng
Chen, Pinzhuo
and
Wen, Chang
2021.
Particle-resolved simulation on viscous flow past random and ordered arrays of hot ellipsoidal particles.
International Journal of Multiphase Flow,
Vol. 142,
Issue. ,
p.
103736.
Chen, Pinzhuo
Chen, Sheng
Yang, Mengmeng
and
Li, Shuiqing
2021.
Falling clouds of particles with finite inertia in viscous flows.
Physics of Fluids,
Vol. 33,
Issue. 3,
Gu, Junping
Wang, Qinggong
Wu, Yuxin
Feng, Lele
Zhang, Guang
Li, Shuiqing
Tian, Lin
and
Yao, Wei
2021.
Numerical study of particle transport by an alternating travelling-wave electrostatic field.
Acta Astronautica,
Vol. 188,
Issue. ,
p.
505.
Hu, Jiawei
Pei, Chunlei
Zhang, Ling
Liang, Cai
and
Wu, Chuan‐Yu
2022.
Numerical analysis of frictional charging and electrostatic interaction of particles.
AIChE Journal,
Vol. 68,
Issue. 5,
Qian, Xiaoyu
Ruan, Xuan
and
Li, Shuiqing
2022.
Effect of interparticle dipolar interaction on pore clogging during microfiltration.
Physical Review E,
Vol. 105,
Issue. 1,
Hu, Jiawei
Liang, Cai
Wu, Chuan-Yu
Wang, Xin
and
Zhou, Qun
2022.
Effect of electrostatic interactions on particle dispersion in a rotating spherical container.
Powder Technology,
Vol. 398,
Issue. ,
p.
117063.
Chen, Sheng
2023.
Microparticle Dynamics in Electrostatic and Flow Fields.
p.
81.
Chen, Sheng
2023.
Microparticle Dynamics in Electrostatic and Flow Fields.
p.
1.
Ma, Zhan
and
Pan, Wenxiao
2024.
Shape deformation, disintegration, and coalescence of suspension drops: Efficient simulation enabled by graph neural networks.
International Journal of Multiphase Flow,
Vol. 176,
Issue. ,
p.
104845.
 $Re_{p}$ and cloud-to-particle size ratio
$Re_{p}$ and cloud-to-particle size ratio 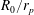 $R_{0}/r_{p}$. A dimensionless charge parameter
$R_{0}/r_{p}$. A dimensionless charge parameter 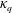 $\unicode[STIX]{x1D705}_{q}$ is constructed to quantify the effect of the repulsion, and a critical value
$\unicode[STIX]{x1D705}_{q}$ is constructed to quantify the effect of the repulsion, and a critical value 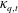 $\unicode[STIX]{x1D705}_{q,t}$ is deduced, which successfully captures the transition of a cloud from hydrodynamically controlled regime to repulsion-controlled regime. Our results also reveal that, with sufficiently strong repulsion, the cloud undergoes a universal self-similar expansion. Scaling laws of cloud radius
$\unicode[STIX]{x1D705}_{q,t}$ is deduced, which successfully captures the transition of a cloud from hydrodynamically controlled regime to repulsion-controlled regime. Our results also reveal that, with sufficiently strong repulsion, the cloud undergoes a universal self-similar expansion. Scaling laws of cloud radius  $R_{cl}$ and particle number density
$R_{cl}$ and particle number density  $n$ are obtained by solving a continuum convection equation.
$n$ are obtained by solving a continuum convection equation.