Article contents
On precessing flow in an oblate spheroid of arbitrary eccentricity
Published online by Cambridge University Press: 05 March 2014
Abstract
We consider a homogeneous fluid of viscosity  $\nu $ confined within an oblate spheroidal cavity of arbitrary eccentricity
$\nu $ confined within an oblate spheroidal cavity of arbitrary eccentricity 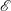 $\mathcal{E}$ marked by the equatorial radius
$\mathcal{E}$ marked by the equatorial radius 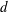 $d$ and the polar radius
$d$ and the polar radius 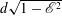 $d \sqrt{1-\mathcal{E}^2}$ with
$d \sqrt{1-\mathcal{E}^2}$ with 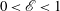 $0<\mathcal{E}<1$ . The spheroidal container rotates rapidly with an angular velocity
$0<\mathcal{E}<1$ . The spheroidal container rotates rapidly with an angular velocity 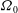 ${\boldsymbol{\Omega}}_0 $ about its symmetry axis and precesses slowly with an angular velocity
${\boldsymbol{\Omega}}_0 $ about its symmetry axis and precesses slowly with an angular velocity 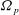 ${\boldsymbol{\Omega}}_p$ about an axis that is fixed in space. It is through both topographical and viscous effects that the spheroidal container and the viscous fluid are coupled together, driving precessing flow against viscous dissipation. The precessionally driven flow is characterized by three dimensionless parameters: the shape parameter
${\boldsymbol{\Omega}}_p$ about an axis that is fixed in space. It is through both topographical and viscous effects that the spheroidal container and the viscous fluid are coupled together, driving precessing flow against viscous dissipation. The precessionally driven flow is characterized by three dimensionless parameters: the shape parameter  $\mathcal{E}$ , the Ekman number
$\mathcal{E}$ , the Ekman number 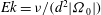 ${\mathit{Ek}}=\nu /(d^2 \delimiter "026A30C {\boldsymbol{\Omega}}_0\delimiter "026A30C )$ and the Poincaré number
${\mathit{Ek}}=\nu /(d^2 \delimiter "026A30C {\boldsymbol{\Omega}}_0\delimiter "026A30C )$ and the Poincaré number 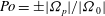 ${\mathit{Po}}=\pm \delimiter "026A30C {\boldsymbol{\Omega}}_p\delimiter "026A30C / \delimiter "026A30C \boldsymbol{\Omega}_0\delimiter "026A30C $ . We derive a time-dependent asymptotic solution for the weakly precessing flow in the mantle frame of reference satisfying the no-slip boundary condition and valid for a spheroidal cavity of arbitrary eccentricity at
${\mathit{Po}}=\pm \delimiter "026A30C {\boldsymbol{\Omega}}_p\delimiter "026A30C / \delimiter "026A30C \boldsymbol{\Omega}_0\delimiter "026A30C $ . We derive a time-dependent asymptotic solution for the weakly precessing flow in the mantle frame of reference satisfying the no-slip boundary condition and valid for a spheroidal cavity of arbitrary eccentricity at 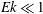 ${\mathit{Ek}}\ll 1$ . No prior assumptions about the spatial–temporal structure of the precessing flow are made in the asymptotic analysis. We also carry out direct numerical simulation for both the weakly and the strongly precessing flow in the same frame of reference using a finite-element method that is particularly suitable for non-spherical geometry. A satisfactory agreement between the asymptotic solution and direct numerical simulation is achieved for sufficiently small Ekman and Poincaré numbers. When the nonlinear effect is weak with
${\mathit{Ek}}\ll 1$ . No prior assumptions about the spatial–temporal structure of the precessing flow are made in the asymptotic analysis. We also carry out direct numerical simulation for both the weakly and the strongly precessing flow in the same frame of reference using a finite-element method that is particularly suitable for non-spherical geometry. A satisfactory agreement between the asymptotic solution and direct numerical simulation is achieved for sufficiently small Ekman and Poincaré numbers. When the nonlinear effect is weak with  $\delimiter "026A30C {\mathit{Po}}\delimiter "026A30C \ll 1$ , the precessing flow in an oblate spheroid is characterized by an azimuthally travelling wave without having a mean azimuthal flow. Stronger nonlinear effects with increasing
$\delimiter "026A30C {\mathit{Po}}\delimiter "026A30C \ll 1$ , the precessing flow in an oblate spheroid is characterized by an azimuthally travelling wave without having a mean azimuthal flow. Stronger nonlinear effects with increasing 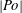 $\delimiter "026A30C {\mathit{Po}}\delimiter "026A30C $ produce a large-amplitude, time-independent mean azimuthal flow that is always westward in the mantle frame of reference. Implications of the precessionally driven flow for the westward motion observed in the Earth’s fluid core are also discussed.
$\delimiter "026A30C {\mathit{Po}}\delimiter "026A30C $ produce a large-amplitude, time-independent mean azimuthal flow that is always westward in the mantle frame of reference. Implications of the precessionally driven flow for the westward motion observed in the Earth’s fluid core are also discussed.
Information
- Type
- Papers
- Information
- Copyright
- © 2014 Cambridge University Press
References
- 17
- Cited by

