Article contents
Nucleation and growth dynamics of vapour bubbles
Published online by Cambridge University Press: 25 November 2019
Abstract
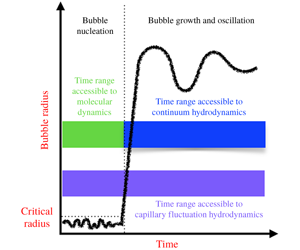
The nucleation of vapour bubbles in stretched or overheated (metastable) liquids is a complex phenomenon with a wide spectrum of applications. Several models, with different levels of detail, have been proposed to predict the key features of bubble dynamics from bubble formation up to its growth, transport and deformation. Most of them focus separately on a few of these aspects. Here, we present a thorough model based on an isothermal diffuse interface description of a two-phase liquid–vapour system endowed with thermal fluctuations, exploiting Landau and Lifshitz’s fluctuating hydrodynamic theory. The stochastic forcing allows for the spontaneous appearance of vapour clusters inside the liquid; the diffuse interface approach provides the hydrodynamic description of the subsequent growth and transport dynamics. In this work we focus on a coarse-grained version of this model, obtained through the averaging of the complete three-dimensional equations on spherical shells: the resulting stochastic equations will spatially depend on the radial distance from the vapour cluster centre. The numerical simulations give access to the mean first passage time, i.e. the time until, on average, the formation of a supercritical bubble. A rough estimate shows that the computational effort is reduced by four orders of magnitude with respect to brute-force atomistic simulations and by two orders of magnitude with respect to the full three-dimensional fluctuating model. The simulations extend up to the very long time scales, allowing us to analyse inertially driven bubble oscillations in confined systems with perfect agreement with available theoretical predictions.
Information
- Type
- JFM Papers
- Information
- Copyright
- © 2019 Cambridge University Press
References
- 55
- Cited by

